If 1 mmole of NaOH is added into a solution in which there is 8 mmoles of aspartic acid with a net -1 charge and 10 moles of aspartic acid with a ned -2 charge, what would the pH of the solution be?

! LONG ANSWER !!

The idea here is that aspartic acid, #"C"_4"H"_7"NO"_4#, is a triprotic acid, which means that it contains three acidic protons that it can release in solution.

For simplicity, I'll use #"H"_3"A"^(+)# as the notation for aspartic acid. Now, these acidic protons will come off at different pH values.

These pH values will depend on the acid's #pK_a# values, which are listed as

#{(pK_(a1) = 1.99), (pK_(a2) = 3.90), (pK_(a3) = 9.90) :}#

http://academics.keene.edu/rblatchly/Chem220/hand/npaa/aawpka.htm

Here's how a molecule of aspartic acid looks like when fully protonated

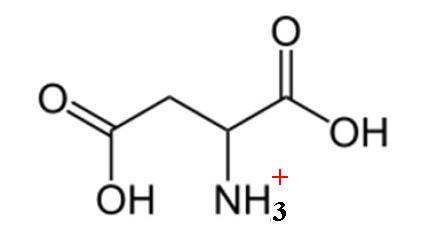
This is the form you get at pH values that are below #pK_(a1)#. Notice that the molecule carries an overall #(color(red)(1+))# charge from the protonated #-"NH"_3^(+)# group.

Here's how a titration would look like for the titration of aspartic acid with a strong base

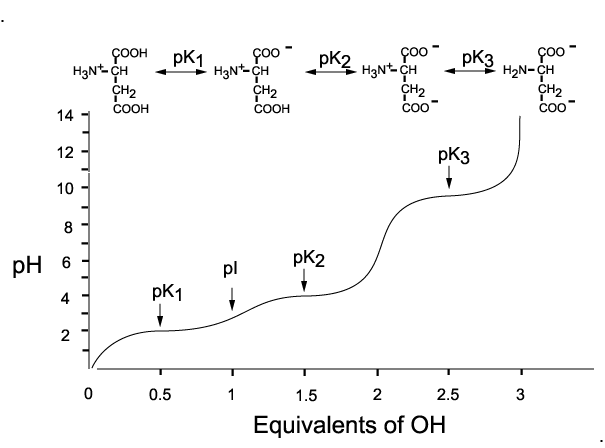
As the pH of the solution increases from #pK_(a1)# to just below #pK_(a2)#, a proton from one of the two carboxyl groups starts to comes off, leaving behind an #alpha#-carboxilic acid group, #"COO"^(-)#.

At this point, the molecule is neutral, since the #(color(red)(1+))# charge on the protonated amino group is balanced by the #(color(red)(1-))# charge on the #alpha#-carboxilic acid group.

The same thing happens as the pH increases from #pK_(a2)# to just below #pK_(a3)# - the proton from the second carboxyl group comes off, leaving behind a second #alpha#-carboxilic acid group.

At this point, the molecule carries a #(color(red)(1-))# charge, since you now get two #(color(red)(1-))# charges and only one #(color(red)(1+))# charge.

Finally, at pH values that exceed #pK_(a3)#, the last acidic proton comes off, leaving behind the amine group, #-"NH"_2#.

At this point, the molecule carries a #(color(red)(2-))# net charge.

So, you know that your solution contains the #"HA"^(-)# and #"A"^(2-)# forms of the molecule. More specifically, it contains #"8 mmoles"# of #"HA"^(-)# and #"10 mmoles"# of #"A"^(2-)#.

Since #"A"^(2-)# is the conjugate base of #"HA"^(-)#, you can say that you're dealing with a buffer solution that contains a weak acid, #"HA"^(-)#, and its conjugate base.

This means that you can use the Henderson - Hasselbalch equation to help you figure out the pH of the solution

#color(blue)("pH" = pK_a + log( (["conjugate base"])/(["weak acid"])))#

Since #"HA"^(-)# is your acidic molecule, you will need to use #pK_(a3)# in the above equation.

Right from the start, you can tell that the pH of the solution before adding the base is higher than #pK_(a3)#, since you have more moles of conjguate base than you have of weak acid.

Now, sodium hydroxide is a strong base that dissociates in a #1:1# mole ratio to produce hydroxide anions, #"OH"^(-)#, in solution.

The hydroxide anions will then neutralize the #"HA"^(-)# molecules to produce water and #"A"^(2-)#, the conjugate base of the acid form.

#"HA"_text((aq])^(-) + "OH"_text((aq])^(-) -> "A"_text((aq])^(2-) + "H"_2"O"_text((l])#

You have #1:1# mole ratios between all the species that are taking part in the reaction.

This means that #"1 mmole"# of hydroxide anions will consume #"1 mmol"# of #"HA"^(-)# and produce #"1 mmol"# of #"A"^(2-)#.

After the reaction is complete, the solution will contain

#n_(OH^(-)) = "0 moles" -># completely consumed by the reaction

#n_("HA"^(-)) = "8 mmoles" - "1 mmole" = "7 mmoles"#

#n_(A^(2-)) = "10 mmoles" + "1 mmole" = "11 mmoles"#

This means that you have

#"pH" = pK_(a3) + log( (["A"^(2-)])/(["HA"^(-)]))#

Since the volume of the buffer is the same for all species, you can use the number of moles in the H - H equation.

Therefore,

#"pH" = 9.90 + log( (11 color(red)(cancel(color(black)("mmoles"))))/(7color(red)(cancel(color(black)("mmoles"))))) = color(green)(10.1)#