How would I write #-4x^2 + 9y^2 + 32x + 36y - 64 = 0# in standard form? What are the steps?

#-4x^2 + 9y^2 + 32x + 36y - 64 = 0# is in the General Cartesian Form of a Conic Section

#Ax^2+Bxy + Cy^2+Dx +Ey + F = 0#

One should use the Discriminant:

#Delta = B^2-4AC#

to determine which conic section the equation describes.

If #Delta < 0#, then it is a circle or an ellipse. If #B = 0# and #A=C# then it is a circle. Otherwise, it is an ellipse.

If #Delta = 0#, then it is a parabola.

If #Delta > 0#, then it is a hyperbola and, if #A+C = 0#, then it is a square hyperbola.

We observe that #A = -4, B = 0, and C = 9#:

#Delta = 0^2-4(-4)(9) = 144 #, therefore, it is a hyperbola.

There are two standard forms for a hyperbola.

1 The horizontal transverse axis form:

#(x-h)^2/a^2-(y-k)^2/b^2=1#

2 The vertical transverse axis form:

#(y-k)^2/a^2-(x-h)^2/b^2=1#

If either case, we need to make the equation fit the patterns:

#(x - h)^2 = x^2-2hx+h^2# and #(y - k)^2= y^2-2ky +k^2#

then one of the two forms will follow.

Returning to the equation:

#-4x^2 + 9y^2 + 32x + 36y - 64 = 0#

Add 64 to both sides and group the x terms and y terms together:

#-4x^2 + 32x + 9y^2 + 36y = 64#

Please observe that the coefficient of the #x^2# term is -4; this means that we need to multiply the pattern for the x terms by -4:

#-4(x - h)^2 = -4x^2+8hx-4h^2#

This tells us that we must subtract #4h^2# from both sides of the equation:

#-4x^2 + 32x-4h^2 + 9y^2 + 36y = 64-4h^2#

We can find the value of h by setting the middle term in the pattern equal to the corresponding term in the equation:

#8hx = 32x#

#h = 4#

We can substitute #-4x^2 + 32x-4h^2= -4(x-4)^2# on the left side and we can substitute #h = 4# on the right:

#-4(x-4)^2 + 9y^2 + 36y = 64-4(4)^2#

Simplify the right side:

#-4(x-4)^2 + 9y^2 + 36y = 0#

Please observe that the coefficient of the #y^2# term is 9; this means that we need to multiply the pattern for the y terms by 9:

#9(y - k)^2= 9y^2-18ky +9k^2#

This tells us that we must add #9k^2# to both sides of the equation:

#-4(x-4)^2 + 9y^2 + 36y +9k^2= 9k^2#

We can find the value of k by setting the middle term in the pattern equal to the corresponding term in the equation:

#-18ky = 36y#

#k = -2#

We can substitute #9y^2 + 36y +9k^2 = 9(y-(-2))^2# on the left and #k = -2# on the right:

#-4(x-4)^2 + 9(y-(-2))^2= 9(-2)^2#

Swap the two terms on the left and simplify the right:

#9(y-(-2))^2-4(x-4)^2 = 36#

Divide both sides of the equation by 36:

#(y-(-2))^2/4-(x-4)^2/9 = 1#

Write the denominators as squares:

#(y-(-2))^2/2^2-(x-4)^2/3^2 = 1#

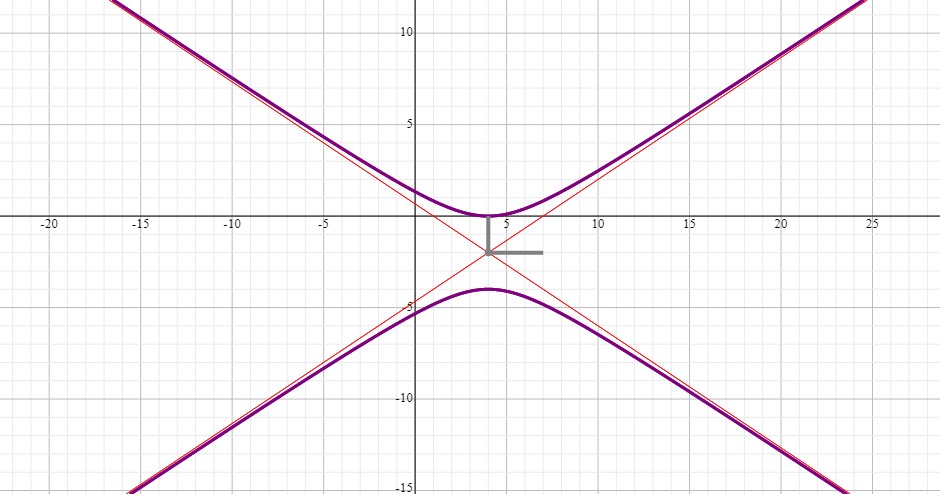
The above is standard form for a hyperbola with a vertical transverse axis.

.

#-4x^2+9y^2+32x+36y-64=0#

First we factor out the coefficient of #x^2# from the #x# terms and coefficient of #y^2# from the #y# terms:

#-4(x^2-8x)+9(y^2+4y)-64=0#

Then we need to complete the square for the #x# and #y# parts by adding and subtracting a constant as such:

#-4(x^2-8x+16-16)+9(y^2+4y+4-4)-64=0#

#-4[(x-4)^2-16]+9[(y+2)^2-4]-64=0#

Now, we multiply the #-4# and #9# through and remove the brackets:

#-4(x-4)^2+64+9(y+2)^2-36-64=0#

After simplifying :

#-4(x-4)^2+9(y+2)^2=36#

Now. we divide the equation by #36#:

#(-4(x-4)^2)/36+(9(y+2)^2)/36=1#

After simplifying, we get:

#(-(x-4)^2)/9+(y+2)^2/4=1#

#(y+2)^2/4-(x-4)^2/9=1#

This is the equation of a hyperbola whose graph can be seen below: