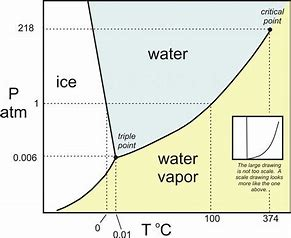
Now, this graph DOES exaggerate the slopes, but pressure is plotted against temperature...and we are interested in the SLOPE of the transition ICE to WATER....(DeltaP)/(DeltaT)...we also introduce the Clapeyron equation, the which says FOR A PHASE CHANGE...
(DeltaP)/(DeltaT)-=(DeltaS)/(DeltaV)
Now for the transition....H_2O(s) rarr H_2O(l)...CLEARLY DeltaS is a POSITIVE QUANTITY. Agreed? The statistical probability of disorder is GREATER in the LIQUID state than in the SOLID STATE. But given the Clapeyron equation, if entropy change is a POSITIVE quantity, DeltaV MUST be a negative quantity in the phase transition...in other words in the liquid state, the density is GREATER than in the solid state. And the result? Well, ICE floats on water, and this is something that we (and the Captain of the Titanic) know full well...
And while this property is not entirely unique to water, it is UNUSUAL and I think this is what the question wants you to consider...

