How does the air resistance affects the graph of a free falling body?
Please show 2 separate graphs in answering your question.
Please show 2 separate graphs in answering your question.
1 Answer
See explanation
Explanation:
The differential equation of the free fall with air resistance is :
with
m = mass of falling object
g = gravity constant = 10 m/s² (9.81 but we take 10 for simplicity)
c = constant depending on the Cx value of the object among others
v = vertical velocity downwards of the falling object in m/s
The solution is
with
and
If we take
we get
with h the height fallen, measured downwards.
We have to compare this with the frictionless fall :
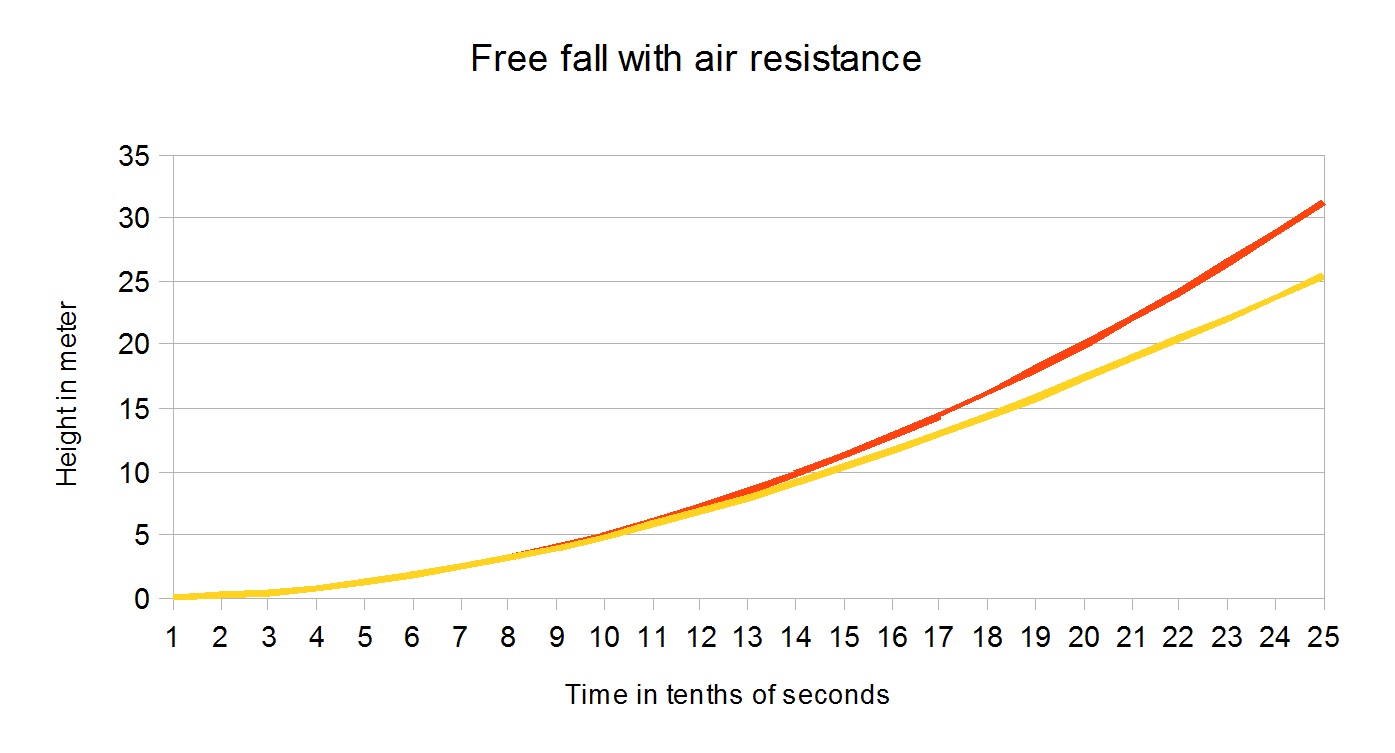
Clearly the latter is a parabole, while the former is flatter.
For the rest there is not much to see about the graphics, but i have added a graph anyway (the red line is frictionless fall, the yellow with air resistance) :