How do you write in simplest radical form the coordinates of point A if A is on the terminal side of angle in standard position whose degree measure is #theta#: OA=15, #theta=135^circ#?

1 Answer
Andy Y.
Nov 17, 2017

The coordinates are #(15/sqrt(2),15/sqrt(2))#

Explanation:

Start by making a reference triangle for your angle.

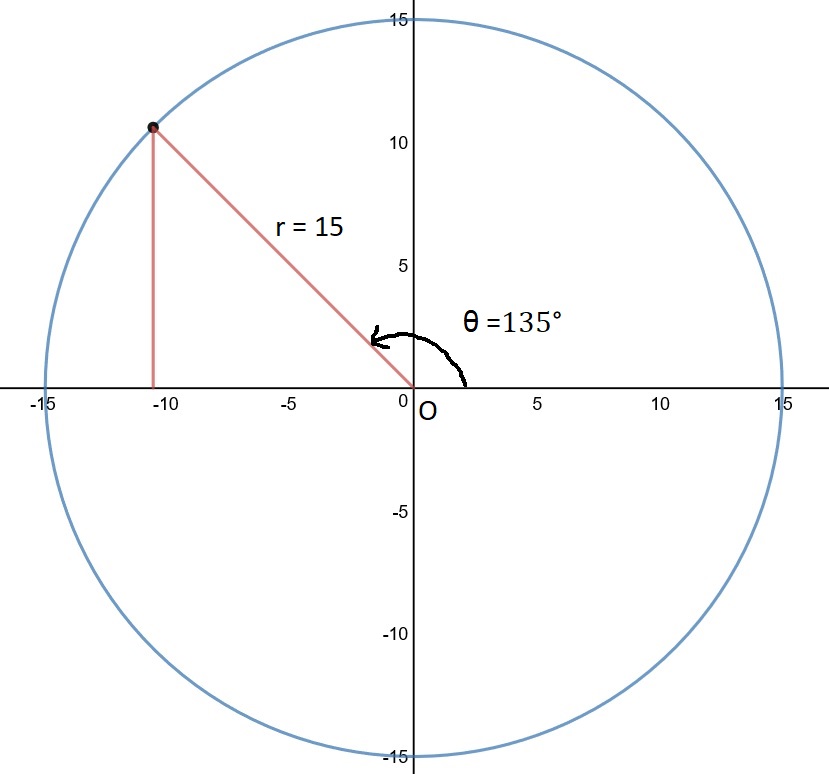
Desmos.com and MS Paint

The inner angle of the right triangle is #180-135=45# degrees. The side opposite the #45# degree angle is the #y# coordinate, and the side adjacent to the #45# degree angle is the #x# coordinate.

The properties of a #45-45-90# right triangle are

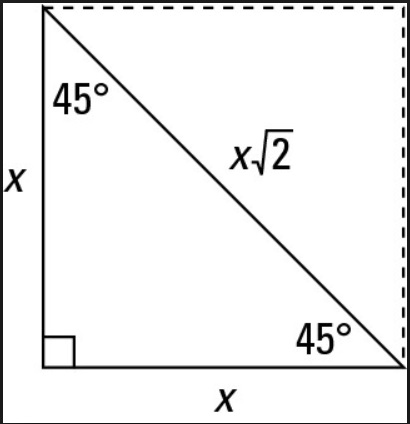
Dummies.com

So the hypotenuse is #15=ysqrt(2)#. We can solve this by dividing both sides by #sqrt(2)#.

#x=15/sqrt(2)# and #y=15/sqrt(2)#.

Thus, the coordinates are #(15/sqrt(2),15/sqrt(2))#

Related questions
See all questions in Radian Measure
Impact of this question
2981 views around the world
You can reuse this answer
Creative Commons License