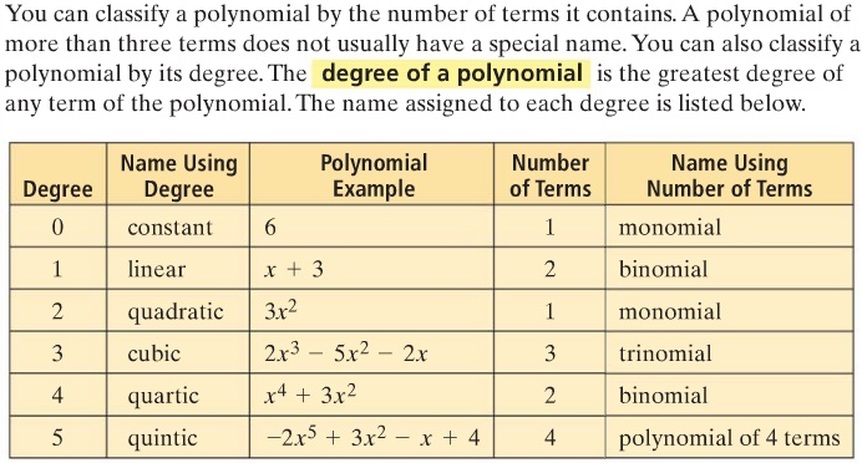
How do you write a polynomial in standard form, then classify it by degree and number of terms $-4p+3p+2p^2$ ?

Question

How do you write a polynomial in standard form, then classify it by degree and number of terms $-4p+3p+2p^2$ ?

1 Answer

Impact of this question

12911 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-12-08T01:20:21

Standard form : $2p^2-p$
Classify by degree: Quadratic
Classify by number of terms: Binomial

Explanation:

Standard form suggests that
- Combine all like terms together
- Rearrange it so that the degrees are arranged in a descending order from left to right.

So in $-4p+3p+2p^2$

We first combine like terms together

$-p+2p^2$

$Note: -4+3=-1, -1p=-p$

Now we rearrange it so the degrees are in descending order

$2p^2-p$

We can see that the degree in $p^2$ is two, so it is classified as a quadratic.

We can also see that there are two terms in this equation, so it is classified as a binomial.
.
.
.

Extra examples:

www.zeihen.com

Science

Math

Humanities

... and beyond

How do you write a polynomial in standard form, then classify it by degree and number of terms $-4p+3p+2p^2$ ?

1 Answer

Explanation:

Related questions

Impact of this question

Science

Math

Humanities

... and beyond

How do you write a polynomial in standard form, then classify it by degree and number of terms -4p+3p+2p^2-4p+3p+2p^2?

1 Answer

Explanation:

Related questions

Impact of this question

How do you write a polynomial in standard form, then classify it by degree and number of terms $-4p+3p+2p^2$ ?