How do you solve #-3x + 5y + z = 10#, #2x + 3y - z = 7#, and #-4x + 2y +3z = -1# using matrices?

Question

5486 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-12-29T18:01:46

x=-1, y=2, z= -3

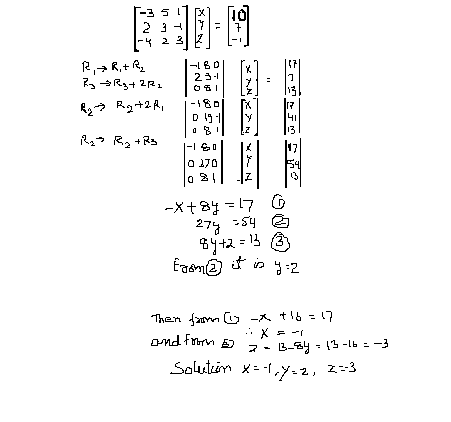
In matrix form, the system can be written as follows and the solved using indicated row operations:

#[(-3,5,1),(2,3,-1),(-4,2,3)][(x),(y),(z)] = [(10),(7),(-1)]#

#R_1 + R_2 to R_1#

#[(-1,8,0),(2,3,-1),(-4,2,3)][(x),(y),(z)] = [(17),(7),(-1)]#

#R_3 + 2R_3 to R_3#

#[(-1,8,0),(2,3,-1),(0,8,1)][(x),(y),(z)] = [(17),(7),(13)]#

#2R_1 + R_2 to R_2#

#[(-1,8,0),(0,19,-1),(0,8,1)][(x),(y),(z)] = [(17),(41),(13)]#

#R_2 - 2R_3 to R_2#

#[(-1,8,0),(0,3,-3),(0,8,1)][(x),(y),(z)] = [(17),(15),(13)]#

#R_2/3 to R_2#

#[(-1,8,0),(0,1,-1),(0,8,1)][(x),(y),(z)] = [(17),(5),(13)]#

#R_3 - 8R_2 to R_3#

#[(-1,8,0),(0,1,-1),(0,0,9)][(x),(y),(z)] = [(17),(5),(-27)]#

#R_3/9 to R_3#

#[(-1,8,0),(0,1,-1),(0,0,1)][(x),(y),(z)] = [(17),(5),(-3)]#

#-1R_1 to R_1#

#[(1,-8,0),(0,1,-1),(0,0,1)][(x),(y),(z)] = [(-17),(5),(-3)]#

#R_3 + R_2 to R_2#

#[(1,-8,0),(0,1,0),(0,0,1)][(x),(y),(z)] = [(-17),(2),(-3)]#

#8R_2 + R_1 to R_1#

#[(1,0,0),(0,1,0),(0,0,1)][(x),(y),(z)] = [(-1),(2),(-3)]#

enter image source here