How do you prove?

Question

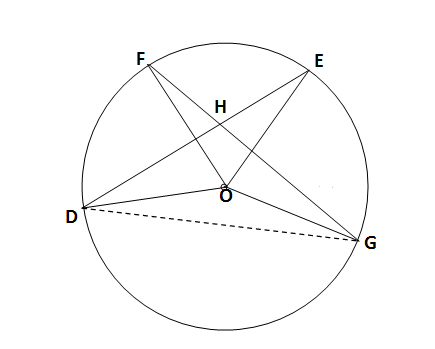
If a circle with centre O has two chords DE and FG which intersect at point H then prove that #/_DOF+/_EOG=2/_EHG#?

1429 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-12-16T10:49:42

Given

A circle with centre O has two chords DE and FG which intersect at point H .

RTP
we are to prove that #/_DOF+/_EOG=2/_EHG#

Construction : D and G are joined.

Proof

Now the central #/_EOG# and peripheral #/_EDG# are on the same arc GE,

So #/_EOG=2/_EDG.....[1]#

Similarly the central #/_DOF# and peripheral #/_FGD# are on the same arc FD,

So #/_DOF=2/_FGD.....[2]#

Adding [1] and [2] we get

#/_DOF+/_EOG=2/_FGD+2/_EDG#

#=2(/_FGD+/_EDG)#

#=2(/_FGD+/_HDG)#

#=2/_EHG#
(exterior angle of a triangle is equal to sum of two of the remote interior angles of the triangle.)