How do you graph #r^2 = sin(2theta)#?

1 Answer
Bio
Sep 11, 2016

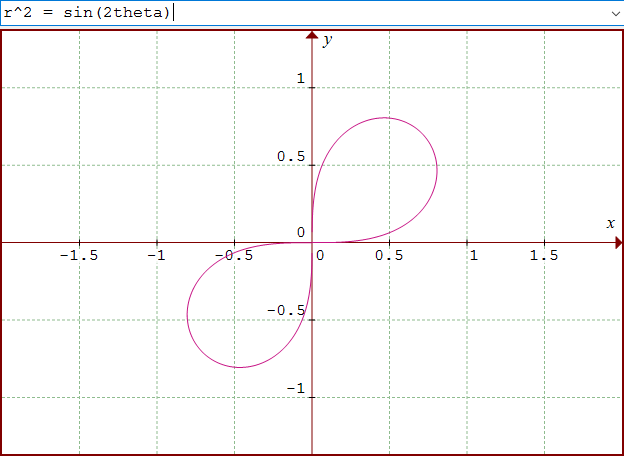
enter image source here

Explanation:

Try plotting with points.

Some easy points would be #(0,0)#, #(pi/12,+-1/sqrt2)#, #(pi/8,+-1/root(4)2)#, #(pi/6,+-root(4)3/sqrt2)#, #(pi/4,+-1)#, #((3pi)/8,+-1/root(4)2)#, #(pi/2,0)#.

From the equation, #sin(2theta)# cannot be negative, so #theta# is restricted to #0 <= theta<= pi/2# or #pi <= theta <= (3pi)/2#.

And most values of #theta# corresponds to a positive and a negative value of #r#, the graph should have rotational symmetry.

If a graphing program for cartesian is available, the cartesian equation is

#(x^2 + y^2)^2 = xy#

Related questions
See all questions in More with Polar Curves
Impact of this question
32134 views around the world
You can reuse this answer
Creative Commons License