How do you graph and solve #|x+6| +8 >2#?

1 Answer
Tony B
Nov 24, 2015

See graph: This may not be the full answer as it was a long time ago that I did these. Hope what I have done helps!

Explanation:

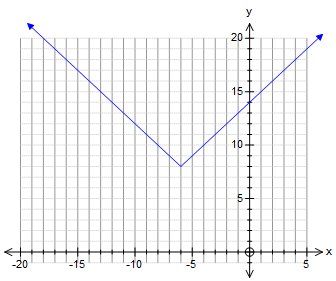
enter image source here

#|x+6| #" is always positive Within the 'absolute' #x# can take any including #-6#. At the point of #x=-6 ; color(white)(...)|x+6| =0# and this is its minimum value. AS you are always adding 8 the minimum value is #0+8=8#. Consequently all value of #y >2#.

Thus all the area above the plot is the feasible region.

Related questions
See all questions in Absolute Value Inequalities
Impact of this question
1384 views around the world
You can reuse this answer
Creative Commons License