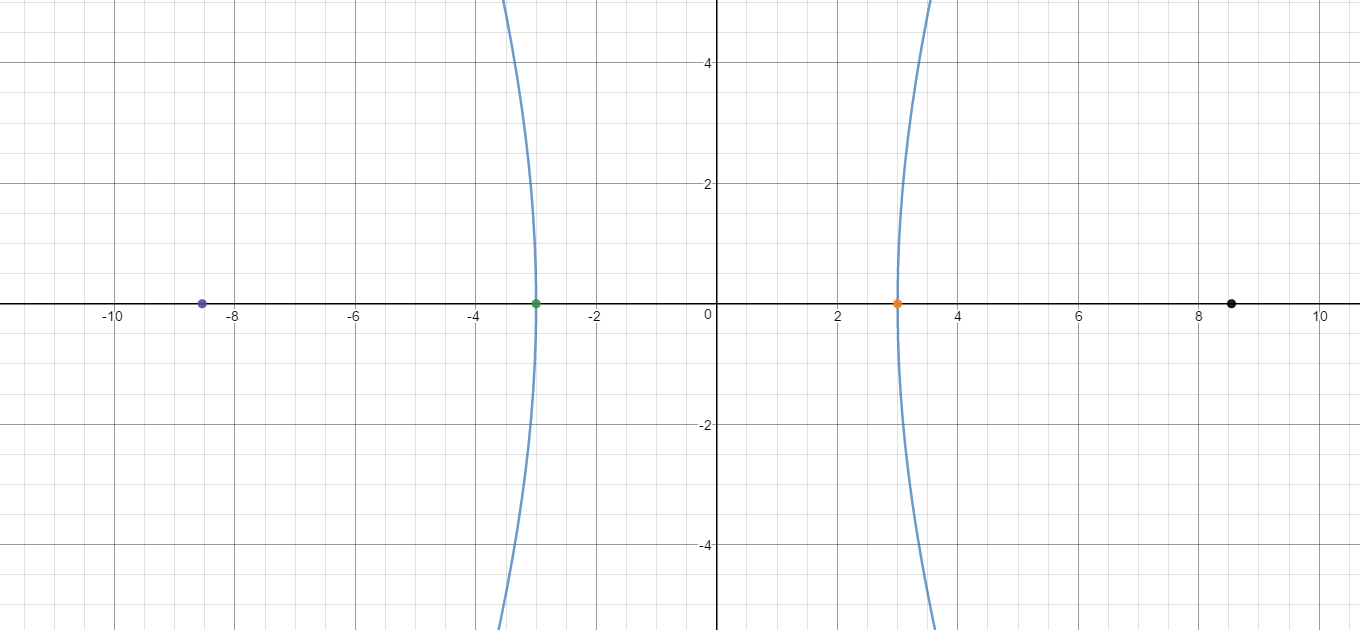How do you find the vertices and foci of #x^2/9-y^2/64=1#?

1 Answer
Douglas K.
Dec 16, 2016

Please read the Hyperbola reference and the explanation (below).

Explanation:

The general form for a hyperbola with a horizontal transverse axis is:

#(x - h)^2/a^2 - (y - k)^2/b^2 = 1" [1]"#

Center: #(h, k)#
Vertices: #(h - a, k) and (h + a, k)#
Foci: #(h - sqrt(a^2 + b^2), k) and (h + sqrt(a^2 + b^2), k)#
Asymptotes: #y = -b/a(x - h) + k and y = b/a(x - h) + k#

Write the given equation in the form of equation [1]:

#(x - 0)^2/3^2 - (y - 0)^2/8^2 = 1" [2]"#

Vertices: #(-3, 0) and (3, 0)#
Foci: #(-sqrt(73), 0) and (sqrt(73), 0)#

Here is a graph with the vertices and the foci.
Desmos.com

Related questions
See all questions in General Form of the Equation
Impact of this question
3189 views around the world
You can reuse this answer
Creative Commons License