How do you find the magnitude and direction of the electric field and magnetic field?
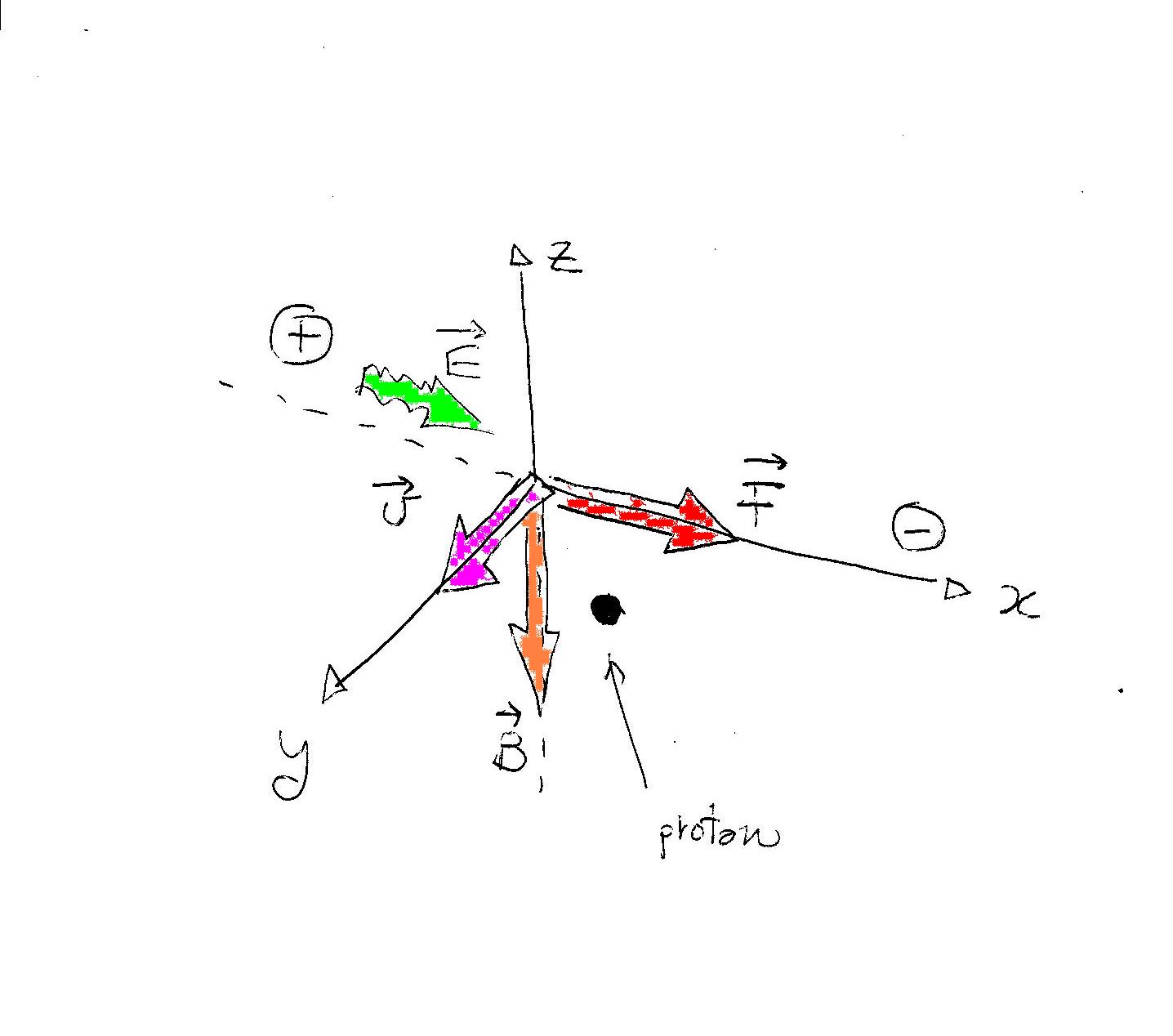
When at rest a pattern experiences a net electromagnetic force of 8.5x10^-13 N pointing in the x-direction. When the proton moves with a speed of 1.5x10^6 m/s in the positive y direction the net electromagnetic force decreases to 7.5x10^-13 N, still pointing in the positive x direction.
When at rest a pattern experiences a net electromagnetic force of 8.5x10^-13 N pointing in the x-direction. When the proton moves with a speed of 1.5x10^6 m/s in the positive y direction the net electromagnetic force decreases to 7.5x10^-13 N, still pointing in the positive x direction.
1 Answer
Have a look if it's understandable (AND if it seems correct!).
Explanation:
Ok, this is complicated...!
I would try using Lorentz Law:
with the vector product:
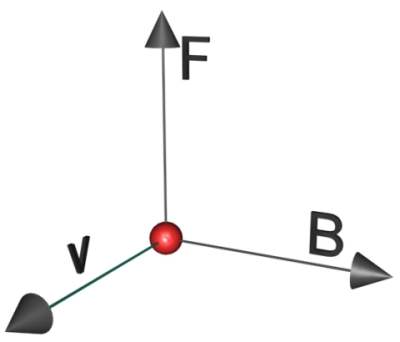
I think in both cases you have a proton (with charge
1) at rest:
(
using moduli (say, along the
along the
2) Use the same approach with the given value of velocity remembering that now you'll have a magnetic field
We need to consider the decrease in force so we need a negative value in the product:
We can write using our data into:
rearranging:
Here it is a possible visual representation: