How do you find the difference of sigma notations?

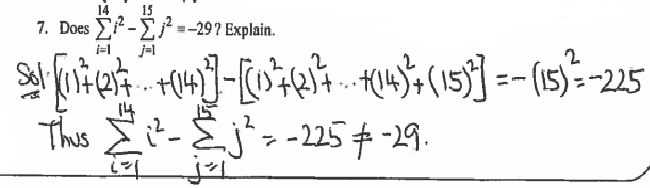
enter image source here

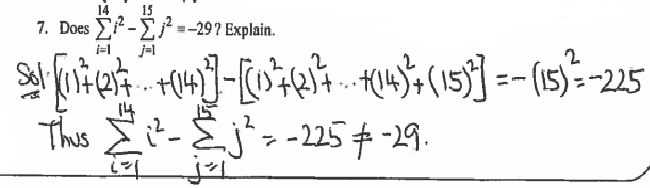
I understand the part where you put (1)^2+(2)^2...+(14)^2 etc... but I don't know how to get the answer as -225. Can someone please list the steps on how to get to -225? Thanks

2 Answers
Somebody N.
Apr 18, 2018

See below.

Explanation:

#sum_(i=1)^(14)i^2-sum_(j=1)^(15)j^2#

Notice that #i^2 and j^2# are one and the same thing. We are therefore just looking for:

#1^2+2^2+3^2+...+14^2+0#

#1^2+2^2+3^2+...+14^2+15^2#

Subtracting:

#-15^2=-225#

AJ
Apr 18, 2018

Well the mistake that you made is while considering the limits of the summation. You cant make operations on the summands of two functions until their lower and upper bound are finite and same. Recall that #sum_(i=1)^kx =sum_(i=1)^ax + sum_(i=a+1)^kx#

so do the same here by altering the limits of summation.
#sum_(i=1)^14i^2-sum_(j=1)^15j^2#
#=sum_(i=1)^14i^2 -{sum_(j=1)^14j^2 + sum_(j=14+1)^15j^2}#
#=sum_(i=1)^14i^2 -sum_(j=1)^14j^2 -sum_(j=15)^15j^2#

Here i and j are nearly the same functions. and their sums are being subtracted so the resultant sum is zero. Let #z=i=j#
so
#=sum_(z=1)^14(i^2-j^2)-sum_(j=15)^15j^2#
#=0-(-15)^2#
#=-225# is the required rectification.

Related questions
Impact of this question
1306 views around the world
You can reuse this answer
Creative Commons License