How do I find the value of sin 5pi / 6?

1 Answer
MrsRudolph221 · bp
Aug 8, 2015

sin #(5pi)/6#= #1/2#

Explanation:

Sin #(5pi)/6#= sin #(pi- pi/6)#= sin #pi/6#= sin 30 = #1/2#

Another way to think about it is to draw the angle in a Unit circle and create the "new" triangle in Quadrant II. teachtogether

Drop a perpendicular to the x-axis and you will have the correct triangle to use.
https://www.google.com/imgres?imgurl=http%3A%2F%2Fcat.ocw.uci.edu%2Fmedia%2FOC08%2F11133%2FOC0111133_Trig2006.gif&imgrefurl=http%3A%2F%2Fcat.ocw.uci.edu%2Foo%2FgetOCWPage.php%3Fcourse%3DOC0811133%26lesson%3D2%26topic%3D2%26page%3D10&docid=AMHSUt2Ajj-ALM&tbnid=9MMwqPn6rIyQHM%3A&vet=1&w=350&h=350&safe=active&bih=560&biw=1096&ved=0ahUKEwjYmJyoiePQAhUaOsAKHebwAuAQMwghKAYwBg&iact=mrc&uact=8

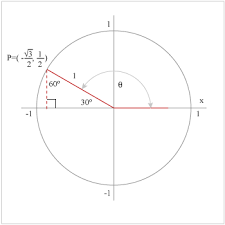From this triangle, you need the opposite leg length, which is #1/2#.
Since the hypotenuse is equal to 1 in the Unit circle, the opposite leg length is the answer for sine. (dividing by 1 is not necessary)

Related questions
See all questions in Trigonometric Functions of Any Angle
Impact of this question
160113 views around the world
You can reuse this answer
Creative Commons License