How can you use trigonometric functions to simplify # 2 e^( ( 4 pi)/3 i ) # into a non-exponential complex number?
1 Answer
Explanation:
Using
#color(blue)" Euler's relationship "#
# re^(itheta) = r( costheta + isintheta ) #
#rArr 2e^((4pi)/3 i) = 2 [(cos((4pi)/3) + isin((4pi)/3)]#
#"----------------------------------------------------------"# now :
#cos((4pi)/3) = -cos(pi/3) # and
# sin((4pi)/3) = -sin(pi/3) # Using
#color(red)" exact value triangle " #
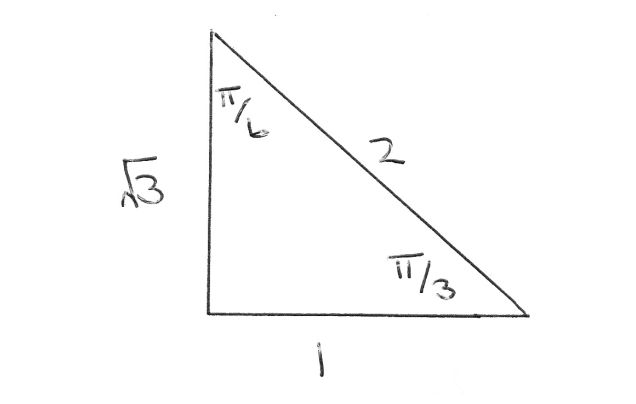
From the triangle we can obtain
#-cos(pi/3) = -1/2" and -sin(pi/3) = -sqrt3/2#
#rArr 2e^((4pi)/3 i )= 2( -1/2 -i sqrt3/2) = -1 - isqrt3 #

