How are the conditions of pressure and temperature shown on a [phase] diagram at which two phases coexist in equilibrium?

Well, luckily for us, phase diagrams are made to be straightforward to read. The curves are literally the lines of two-phase coexistence.

They indicate one degree of freedom (i.e. parallel to the curve at each instantaneous displacement), in accordance with the Gibbs' phase rule.

Consider the phase diagram of water:

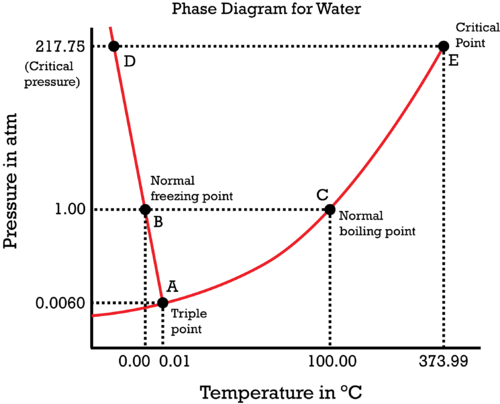
If you are unsure how many phases there are in a given region on a phase diagram, refer to the Gibbs' phase rule to check:

#bb(f = c - p + 2)#

• #f# is the number of degrees of freedom, i.e. the number of coordinate directions you are allowed to move in a phase diagram without moving out of the bounds of consideration.
• #c# is the number of components in the substance (could be a solution full of electrolytes... could be interacting).
• #p# is the number of phases.

Below are example calculations for cases we should already be able to verify physically.

TEST CHECK 1: TRIPLE POINT

For example, consider the triple point of water at #0.01^@ "C"# and #"0.0060 atm"#.

• #f = 0#, because you can't move around in a triple point in any way, unless you are no longer at the triple point.
• #c = 1# for pure substances, because there is only one of itself in itself.

This indicates that the number of phases at a triple point is:

#bb(p) = c + 2 - f = 1 + 2 - 0#

#= bb3# phases in equilibrium at the triple point.

And in fact, that's why it's called a triple point, because three phases coexist at a triple point.

TEST CHECK 2: COEXISTENCE CURVE

Or, consider the liquid-vapor coexistence curve, #bar(AE)#. We should expect TWO coexisting phases: liquid and vapor. Thus, we expect #p = 2#. We have:

• #f = 1#, because if you move parallel to the curve for each instantaneous displacement, you won't move off the curve.
• #c = 1#, because our water sample is assumed to be a pure substance.

And we get:

#bb(p) = c + 2 - f = 1 + 2 - 1#

#= bb(2)# phases in equilibrium with each other, as expected.

TEST CHECK 3: SINGLE-PHASE REGION

Or, consider just being in a single-phase region, like at #50^@ "C"# and #"1.00 atm"#. We should just have one phase: the liquid. We have:

• #f = 2#, because you can move in spirals if you wanted, which requires hybridizing two particular coordinate directions, while still staying in the same two-dimensional region.
• #c = 1#, and we know why at this point.

So,

#bb(p) = c + 2 - f = 1 + 2 - 2#

#= bb(1)# phase exists by itself, which we already said... it's the liquid phase.