Is it possible for acids to have a #"pH"# above #7#? If so, under what circumstances would that be?

Acidity is a measure of the #sf(H^+)# concentration in a solution. A convenient measurement is to use is the pH scale which makes the wide range of numbers involved easier to handle.

#sf(pH=-log[H^+])#

This means that #sf([H^+]=10^(-pH))#

Therefore solution of #sf([H^+]=10^(-2)color(white)(x)"mol/l")# has a pH of 2.

The relationship between pH and concentration is shown on the graphic:

A typical high school textbook will show a pH scale as going from 0 to 14.

A solution of pH 7 is said to be neutral. If the pH is less than 7 then the solution is acidic. If the pH is greater than 7 the solution is an alkali.

This works well under normal laboratory conditions but does not apply generally.

We need to consider the auto - ionisation of water:

#sf(H_2O_((l))rightleftharpoonsH_((aq))^(+)+OH_((aq))^-)#

For which:

#sf(K_w=[H_((aq))^+][OH_((aq))^-]=1.00xx10^(-14)color(white)(x)"mol"^2."l"^(-2))# at #sf(25^@C)#

In pure water #sf([H_((aq))^+]=[OH_((aq))^-])#

#:.##sf([H_((aq))^+]^2=1.00xx10^(-14))#

#sf([H_((aq))^(+)]=sqrt(1.00xx10^(-14))=1.00xx10^(-7)color(white)(x)"mol/l")#

So to get the pH:

#sf(pH=-log(1.00xx10^(-7))=7)#

This gives us our neutral point.

The problem here is that this refers to standard conditions i.e a temperature of #sf(25^@C)#. We know that the temperature can affect the value of #sf(K_c)# and this is such an example.

The auto - ionisation of water is a bond breaking process so is endothermic:

#sf(H_2O_((l))rightleftharpoonsH_((aq))^(+)+OH_((aq))^-)#

#sf(DeltaH)# is +ve.

If we raise the temperature, Le Chatelier's Principle would predict that the position of equilibrium would shift to the right. This would lead to greater dissociation thus increasing the value of #sf(K_w)#.

This has been measured for several temperatures. At #sf(40^@C)# the value of #sf(K_w=2.916xx10^(-14)color(white)(x)"mol"^2."l"^(-2))#

What would happen to the pH ?

#sf([H_((aq))^+]^2=2.916xx10^(-14))#

#:.##sf([H_((aq))^+]=sqrt(2.916xx10^(-14))=1.707xx10^(-7)color(white)(x)"mol/l")#

#:.##sf(pH=-log(1.797xx10^(-7))=6.77)#

This shows that the neutral point has now dropped to pH 6.77.

Using data provided by ChemguideUK I have produced the following chart showing the variation of pH with temperature for pure water:

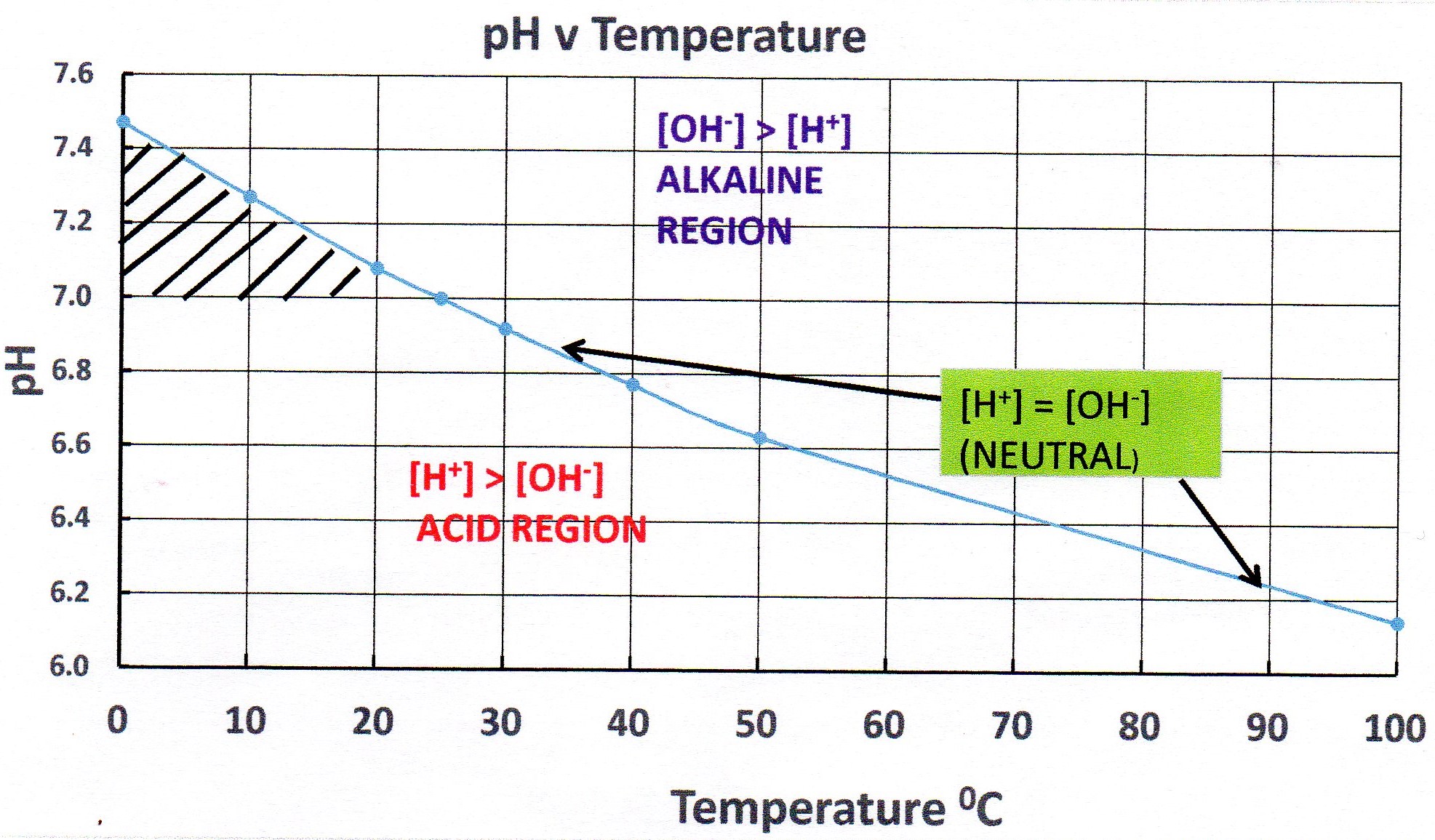
As you can see, the pH falls as the temperature rises. This does not mean that the water has become more acidic. All along the blue line the water is neutral.

This is because #sf([H_((aq))^+]=[OH_((aq))^(-)])#. This is the criteria for neutrality.

Any solution lying below the blue line is in the acidic region of the graph. This is because #sf([H_((aq))^(+)]>[OH_((aq))^-])#

Any solution lying above the blue line is in the alkaline region of the graph. This is because #sf([OH_((aq))^(-)]>[H_((aq))^+])#.

Returning to the original question, you can see any solution lying in the black hatched region can have a pH greater than 7 yet is acidic.

Conversely, you can see that at higher temperatures you can have alkaline solutions whose pH is less than 7.

In summary, the criteria for a neutral solution is not necessarily that pH = 7, rather it is that #sf([H_((aq))^+]=[OH_((aq))^-])#

By the same argument an alkaline solution does not necessarily have a pH > 7, it is when #sf([OH_((aq))^(-)]>[H_((aq))^+])#.

Finally, an acidic solution is not necessarily where pH < 7, it is when #sf([H_((aq))^(+)]>[OH_((aq))^-])#