Differentiate #\frac{r}{\sqrt{r^2+5}}# ?

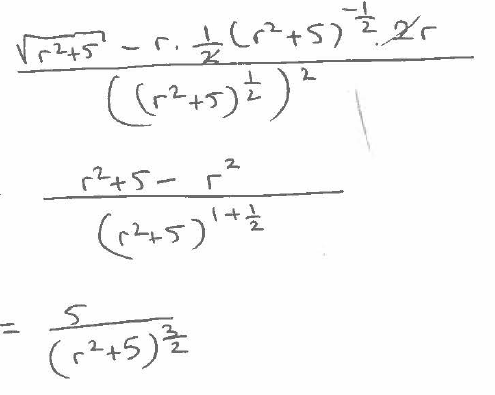
enter image source here

I'm unsure how they went from the first step to the second step? How did the #(r^2+5)^(1/2)# on the denominator from the first step turn into an exponent of 1 on the second step? Also, how do you get the -r^2 in the numerator in the second step?

2 Answers
Jim G.
Jun 12, 2018

#5/(sqrt((r^2+5)^3)#

Explanation:

#"differentiate using the "color(blue)"product rule"#

#"given "y=g(h(x))" then"#

#dy/dx=g(x)h'(x)+h(x)g'(x)larrcolor(blue)"product rule"#

#"here "y=r/(sqrt((r^2+5)^(1/2)))=r(r^2+5)^(-1/2)#

#g(r)=r rArrg'(r)=1#

#h(r)=(r^2+5)^(-1/2)#

#h'(r)=-1/2(r^2+5)^(-3/2)xxd/(dr)(r^2+5)#

#color(white)(h'(x))=-1/2(r^2+5)^(-3/2)xx2r=-r(r^2+5)^(-3/2)#

#(dy)/(dr)=-r^2(r^2+5)^(-3/2)+(r^2+5)^(-1/2)#

#color(white)((dy)/(dr))=(r^2+5)^(-3/2)[cancel(-r^2)cancel(+r^2)+5]#

#color(white)((dy)/(dr))=5/(r^2+5)^(3/2)#

Odlisss
Jun 12, 2018

By expanding by #sqrt(r^2+5)/sqrt(r^2+5)#

Explanation:

Your first question can be answered by a simple algebra rule. We know that :

#(a^m)^n = a^(m*n)#

so therefore #((r^2+5)^(1/2))^2 = (r^2+5)^(2*1/2) = (r^2+5)#

In the next step, we expanded the fraction by #sqrt(r^2+5)/sqrt(r^2+5)#.

Therefore in the denominator we got #(r^2+5)^(3/2)# and the second part of the numerator became :

#-r^2*(r^2+5)^(-1/2)*(r^2+5)^(1/2) = -r^2#.

Related questions
Impact of this question
1672 views around the world
You can reuse this answer
Creative Commons License