Are octonions numbers?

The Octonions are sometimes called the "Cayley Algebra" and sometimes the "Cayley Numbers".

They are an extension of the Real numbers, closed under addition, multiplication, additive inverse and multiplicative inverse of non-zero elements. Addition of octonions is commutative and associative. Multiplication is generally neither commutative nor associative. They have no zero divisors. That is, if #x# and #y# are non-zero octonions then so is #xy#.

Octonions are an extension of the Quaternions, which are an extension of the Complex numbers, which are an extension of the Real numbers.

But what do we mean by calling algebraic objects numbers and why might the octonions qualify?

In the following table are some properties of the real numbers #RR#, complex numbers #CC#, quaternions #bb"H"#, octonions #bb"O"# and sedenions #bb"S"#:

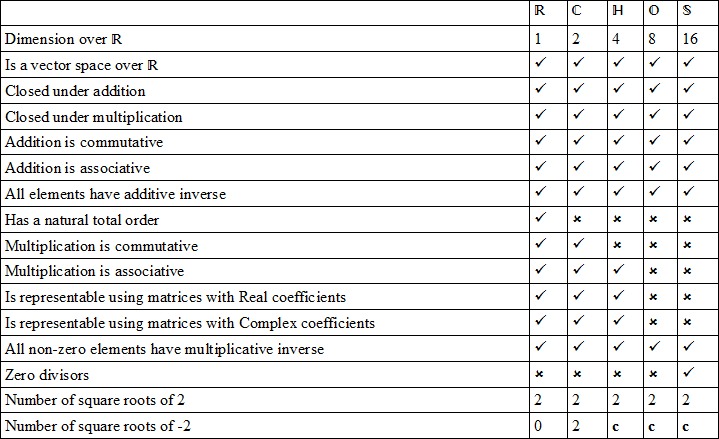
Note that #bb"c"# in the last row of this table is the transfinite number representing the number of Real numbers. That is, there are as many square roots of #-2# in #bb"H"#, #bb"O"# and #bb"S"# as there are Real numbers.

So Real numbers are well behaved and totally ordered, but algebraically incomplete in that they contain no solution to #x^2+1 = 0#

Complex numbers are well behaved and algebraically complete, but have no natural ordering. A polynomial of degree #n# with Complex coefficients has exactly #n# zeros counting multiplicity. Any complex number can be written in the form #a+bi# where #a, b in RR#.

Quaternions have non-commutative multiplication, but are otherwise well behaved. Most polynomials will have an infinite number of quaternion valued zeros. Quaternions are useful for modelling 3 dimensional mechanical systems. Any quaternion can be written in the form #a+bi+cj+dk# where #a, b, c, d in RR#.

Octonions drop the requirement that multiplication be associative. Octonion multiplication does satisfy a weaker condition expressed as saying that multiplication is alternative. Octonions have applications in string theory, special relativity and quantum logic. Any octonion can be written in the form #a_0 e_0 + a_1 e_1 + a_2 e_2 +...+ a_7 e_7# where #e_0 = 1#, #e_1,..,e_7# are imaginary units, and #a_0,...,a_7 in RR#.

To multiply octonions, you can use distributivity of multiplication over addition and the following multiplication table for the units #e_0, e_1,.., e_7#:

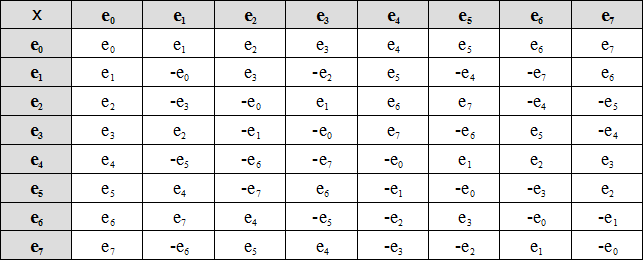
The octonions can be further extended using the Cayley-Dickson construction to form the sedenions - a 16 dimensional algebra over the Reals. Multiplication of sedenions is not even alternative and sedenions have zero divisors. The sedenions seem to have limited use, but one or two interesting properties.

My vote is that Octonions deserve to be called numbers, since they have fairly well defined arithmetic properties and multiple physical applications.