An isosceles triangle has sides A, B, and C, such that sides A and B have the same length. Side C has a length of #4 # and the triangle has an area of #128 #. What are the lengths of sides A and B?

1 Answer
Tom M.
Nov 11, 2017

#A=B=10sqrt41#

Explanation:

When in doubt, draw a diagram.

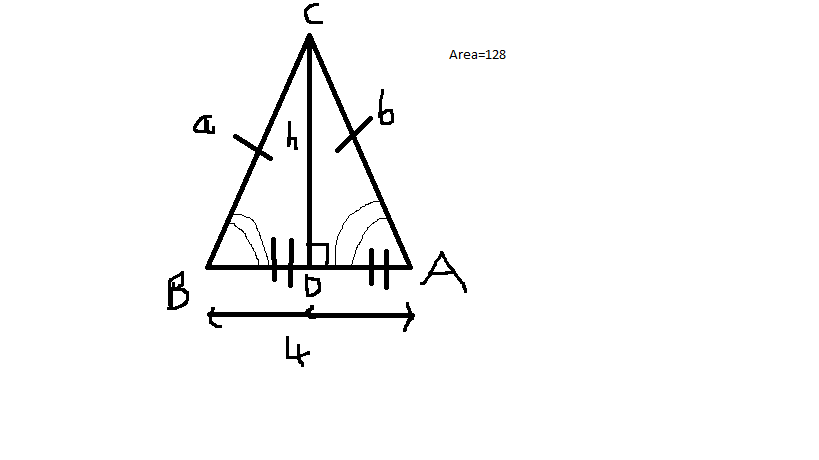
enter image source here
#h# is the perpendicular bisector of #AB# from #C#, and meets #AB# at point #D#.

Sidenote: I have labeled this diagram according to the convention, with angles #A, B# and #C# opposite sides #a, b# and #c# respectively. So where you have #A# and #B#, I have #a# and #b#. Just a note.

We know that #Area=1/2xxbasexxheight#. In here, the base is length 4, and the height is #h#.

#128=1/2xx4h#
#128=2h#
#h=64#

Now, focus just on #triangleBCD# (or #triangle ACD#). To work out the remaining length #a# (and #b=a# since the #triangle# is isosceles) we need to use the Pythagorean Theorem. We have a base of 2, since it is half of the length 4 (remember that #h# is a bisector).

#2^2+64^2=a^2#
#a^2=4100#
#a=sqrt4100=10sqrt41#

Convert back to the format given in the question:

#A=B=10sqrt41#

Related questions
See all questions in Pythagorean Theorem
Impact of this question
1361 views around the world
You can reuse this answer
Creative Commons License