A triangle has sides A, B, and C. The angle between sides A and B is #pi/6# and the angle between sides B and C is #pi/12#. If side B has a length of 9, what is the area of the triangle?

1 Answer
Roella W.
Jan 27, 2016

#A = 1/2B*B*(tan(pi/12)tan(3/4pi))/(tan(3/4pi) + tan(pi/12)#

Explanation:

The area of a triangle is given by #A=1/2Bh# where #B# is the base and #h# is the perpendicular height.
Sketch - not accurate but is useful for the labels.

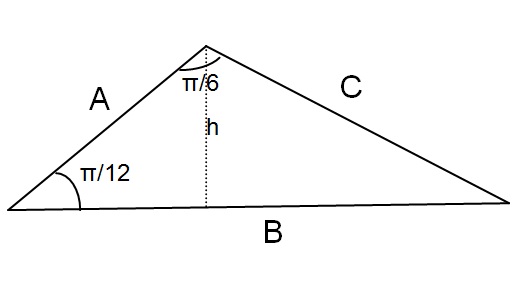
We also know that the sum of the angles in a triangle is #180^o = pi#

The angle between #B# and #C# is therefore #(pi - pi/12 - pi/6)#
#=(12pi-pi-2pi)/12) = 9pi/12 =(3pi)/4#

If #h# intersects with #B# so that #x +y = B# then
#h/(B-y) = tan(pi/12)# and #h/y = tan(3/4pi)#

Then # y=h/tan(3/4pi)#
#:. h = (B-h/tan(3/4pi))tan(pi/12)#

#h = Btan(pi/12) - htan(pi/12)/tan(3/4pi)#

So #h(1+tan(pi/12)/tan(3/4pi)) = Btan(pi/12)#

#h((tan(3/4pi) + tan(pi/12))/tan(3/4pi)) = Btan(pi/12)#

#:. h = B(tan(pi/12)tan(3/4pi))/(tan(3/4pi) + tan(pi/12)#

So the area #A = 1/2B*B(tan(pi/12)tan(3/4pi))/(tan(3/4pi) + tan(pi/12)#

Related questions
See all questions in Area of a Triangle
Impact of this question
1431 views around the world
You can reuse this answer
Creative Commons License