A triangle has sides A, B, and C. Sides A and B are of lengths #8# and #1#, respectively, and the angle between A and B is #(5pi)/8 #. What is the length of side C?

1 Answer
Andrew I.
Jan 27, 2018

#C=8.4334#

Explanation:

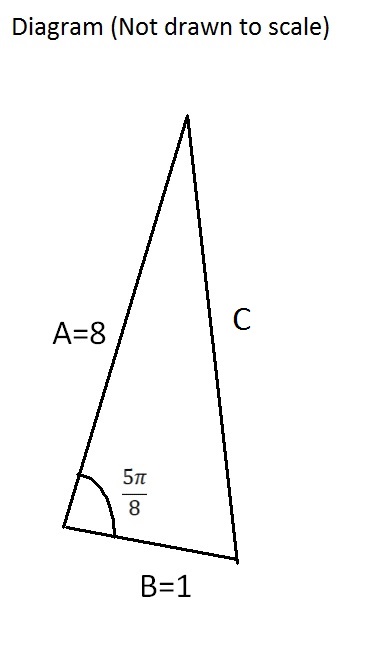
Edited from Microsoft Publisher and Paint

We have two lengths and the angle between them. As such, to determine length C (the opposite length) we must use the law of cosines, in our case that will be:

#C^2=A^2+B^2-2ABcos(x)#

where #x# is the angle made between #A# and #B#.

Plugging in the numbers gives us:

#C^2=8^2+1^2-2(8)(1)cos((5 pi)/8)#

#=65-16(-0.382683)#

#=65+6.12293=71.1229#

So:

#C^2=71.1229#

#therefore C = sqrt(71.1229)=8.4334#

Related questions
See all questions in The Law of Cosines
Impact of this question
1479 views around the world
You can reuse this answer
Creative Commons License