A right triangle has sides A, B, and C. Side A is the hypotenuse and side B is also a side of a rectangle. Sides A, C, and the side of the rectangle adjacent to side B have lengths of #13 #, #4 #, and #3 #, respectively. What is the rectangle's area?

Question

1873 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-04-11T05:05:45

#9sqrt17#

Consider the diagram

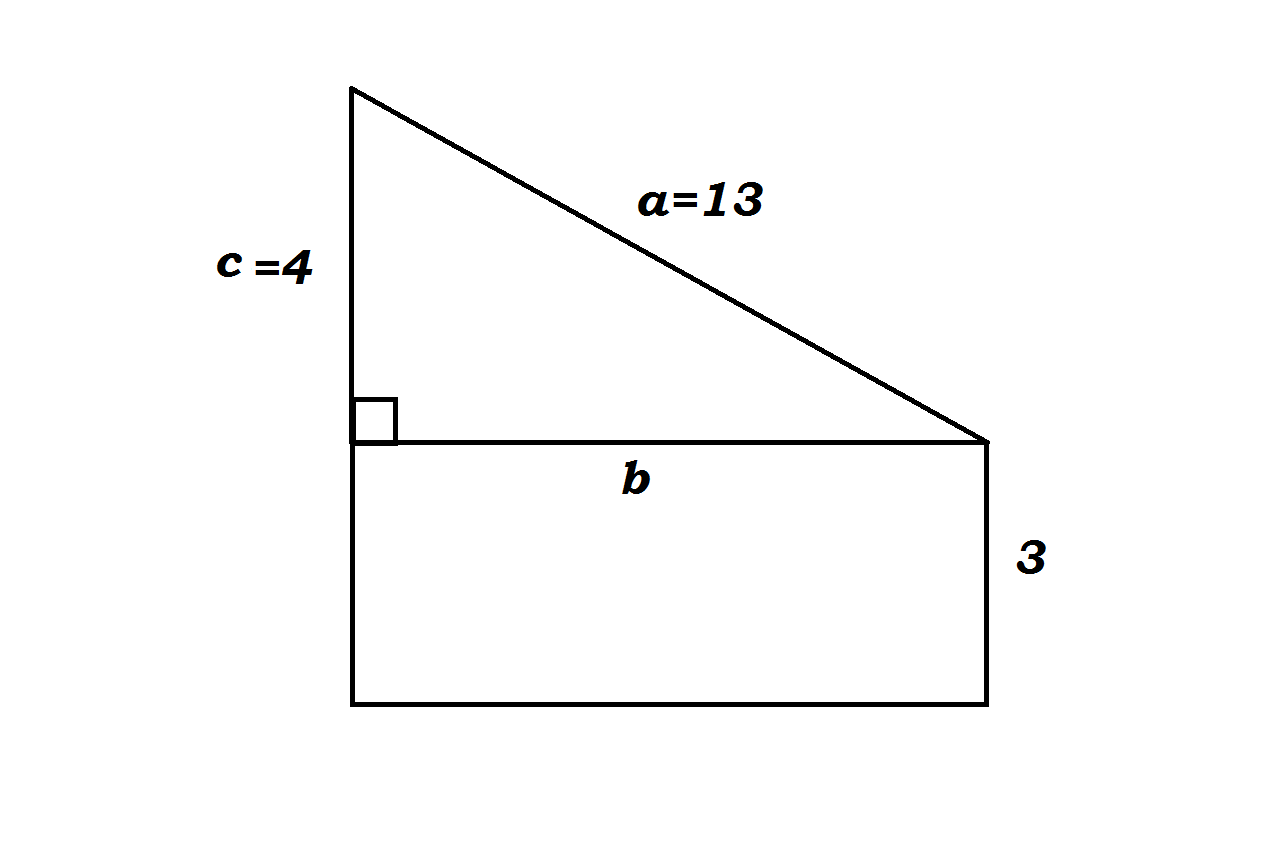
enter image source here

We can find the length of #b# using the Pythagoras-theorem

#color(blue)(a^2+b^2=c^2#

Where,

#aand b# are the right-containing sides and #c# is the Hypotenuse (longest side of a right-triangle)

But,

In this case,#a# is the Hypotenuse and #band c# are the right-containing sides

So,

#rarrb^2+4^2=13^2#

#rarrb^2+16=169#

#rarrb^2=169-16#

#rarrb^2=153#

#rarrb=sqrt153#

#color(green)(rArrb=sqrt153=sqrt(9*17)=3sqrt17#

Now we need to find the area of the rectangle

Area=#color(purple)(l*b#

Where,

#l=l##eng##th,b=breadth#

#color(violet)(l=3sqrt17,b=3#

#rarrArea=3sqrt17*3#

#color(green)(rArr9sqrt17#