A right triangle has sides A, B, and C. Side A is the hypotenuse and side B is also a side of a rectangle. Sides A, C, and the side of the rectangle adjacent to side B have lengths of #15 #, #8 #, and #7 #, respectively. What is the rectangle's area?

2 Answers
Leland Adriano Alejandro
Mar 25, 2016

Area of the rectangle #=7*sqrt161=88.82" "#square units

Explanation:

We can compute for side #b#
because by Pythagorean Theorem

#a^2=b^2+c^2#

#15^2=b^2+8^2#

#b^2=225-64#

#b=sqrt161#

Compute now for the area of the rectangle

Let #x=7# be the length of the other side of the rectangle

Area #=b*x#

Area #=7*sqrt161#

Area #=88.82#

God bless....I hope the explanation is useful.

Veddesh #phi#
Mar 25, 2016

#88.8# #units#

Explanation:

Consider the diagram

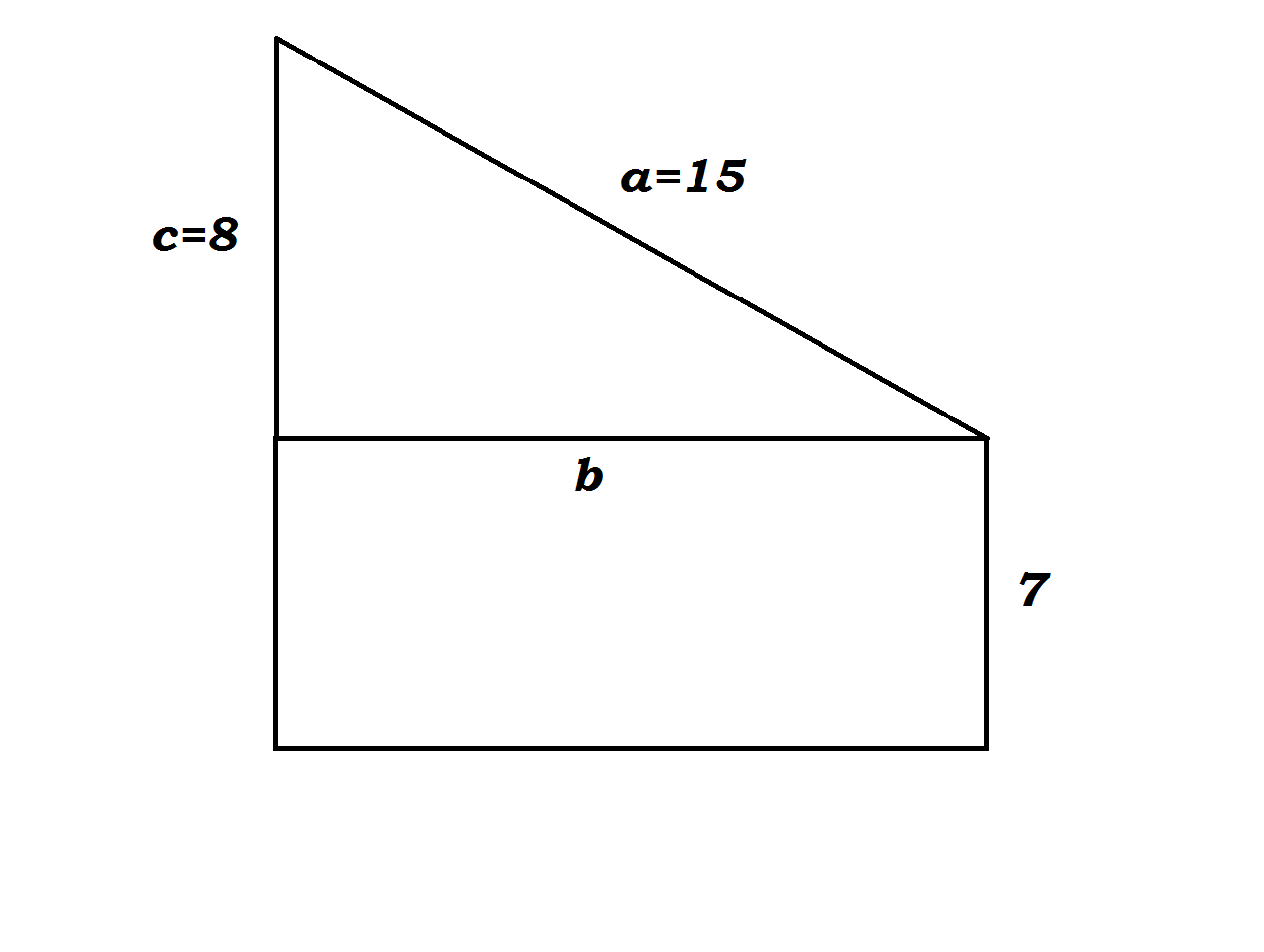
enter image source here

Use the Pythagorean theorem to find the length of #b#

#color(blue)(a^2+b^2=c^2#

Where

#color(red)(a=c,b=b,c=a#

A little confusion! yeah

Remember like this

The square of Hypotenuse of a right triangle equals the sum of the squares of the other two sides

#rarr8^2+b^2=15^2#

#rarr64+b^2=225#

#rarrb^2=225-64#

#rarrb^2=161#

#rArrb=sqrt161#

Now we need to find the area of the rectangle

Area of rectangle

#color(blue)(l*b# #units#

#l=l##e##ng##t##h,b=breadth#

Where

#color(red)(l=sqrt161,b=7#

#:.color(green)(Area=7sqrt161~~88.89#

Related questions
See all questions in Pythagorean Theorem
Impact of this question
1676 views around the world
You can reuse this answer
Creative Commons License