A right triangle has sides A, B, and C. Side A is the hypotenuse and side B is also a side of a rectangle. Sides A, C, and the side of the rectangle adjacent to side B have lengths of #7 #, #6 #, and #15 #, respectively. What is the rectangle's area?

1 Answer
sente
Feb 18, 2016

#15sqrt(13)#

Explanation:

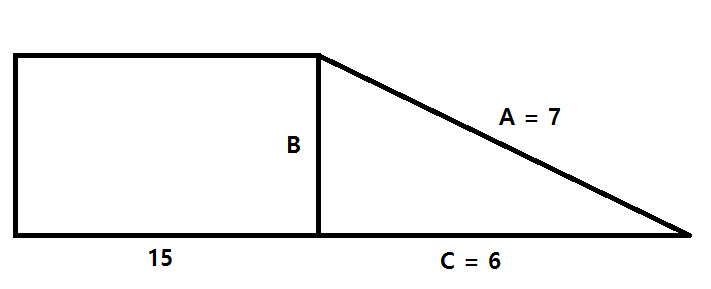
enter image source here

The above (not to scale) picture contains the information given in the problem. In many geometry problems, drawing a picture is a good way to start.

The area of the rectangle is the product of the lengths of its sides, in this case #15B#. To solve for #B#, we can use the Pythagorean theorem, which states that the square of the length of the hypotenuse of a right triangle is equal to the sum of the squares of its legs. In this case, that translates to #A^2 = B^2 + C^2#

Substituting in the given values for #A# and #C#, we obtain

#49 = B^2 + 36#

#=> B^2 = 13#

#=> B = sqrt(13)#

Thus the area of the rectangle is #15sqrt(13)#

Related questions
See all questions in Pythagorean Theorem
Impact of this question
1528 views around the world
You can reuse this answer
Creative Commons License