A projectile is shot from the ground at an angle of #pi/4 # and a speed of #3 /5 m/s#. Factoring in both horizontal and vertical movement, what will the projectile's distance from the starting point be when it reaches its maximum height?

Question

1340 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-02-17T22:45:09

Consider,

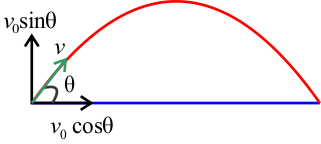physicscatalyst.com

where #theta = pi/4 = 45°# in this case.

Moreover, recall that #nu = 0# at the maximum of the parabolic movement.

#nu = nu_0 + at#
#=> t = (nu - nu_0)/g#

#therefore t = (0 - (0.6m)/ssin(45°))/((-9.8m)/s^2) approx 4.32*10^-2s#

Now: let's understand the horizontal displacement of the projectile. Recall,

#Deltax = nu_0costheta * t#

Hence,

#Deltax = (0.6m)/scos(45°) * 4.32*10^-2s approx 2.60*10^-2m#

This is fairly reasonable, #(0.6m)/s# is very slow.