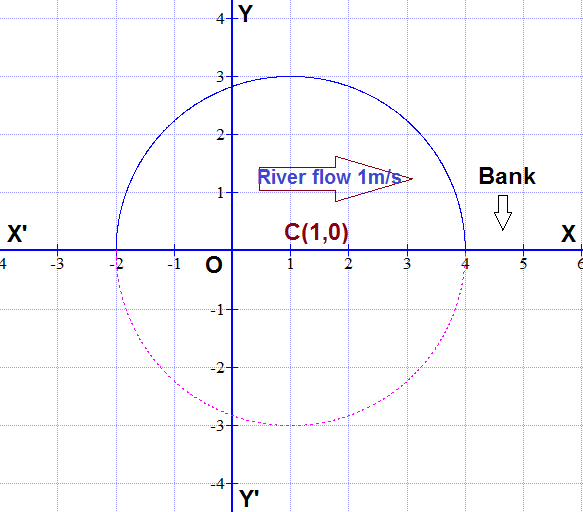
A man can swim in still water with a speed of 3 m/s. #x# and #y# axes are drawn along and normal to the bank of the river flowing to right with a speed of 1 m/s. The man starts swimming from origin #O# at #t=0# second. Assume size ............?

A man can swim in still water with a speed of 3 m/s. #x# and #y# axes are drawn along and normal to the bank of the river flowing to right with a speed of 1 m/s. The man starts swimming from origin #O# at #t=0# second. Assume size of man to be negligible. Find the equation of locus of all possible points where man can reach at #t=1 sec#?

1 Answer
P dilip_k
Dec 29, 2017

enter image source here

Suppose the swimmer swims with velocity #3# m/s in the direction making an angle #theta# with the bank i.e positive direction of X-axis,OX

So the velocity components of the swimmer will be

#V_(OX)=3costheta#

and

#V_(OY)=3sintheta#

As the river is flowing along #OX#

Net velocity along #OX# will be #3costheta+1#

These two velocities are independent on each other as they are orthogonal. The swimmer starts at origin #O# If the displacement of the swimmer after 1 sec along X-axis and Y- axis be #x and y# respectively then

#x=3costheta +1......[1]#
and
#y=3sintheta.............[2]#

From [1] and [2] we get

#(x-1)^2+y^2=3^2cos^2theta+3^2sin^2theta=9#

So equation of locus of all possible points where man can reach at t=1sec will be

#color(magenta)((x-1)^2+y^2=3^2)#, the possible positions are on the blue semicircular line of radius 3m and center #C(1,0)# as shown in figure above.

Related questions
Impact of this question
5370 views around the world
You can reuse this answer
Creative Commons License