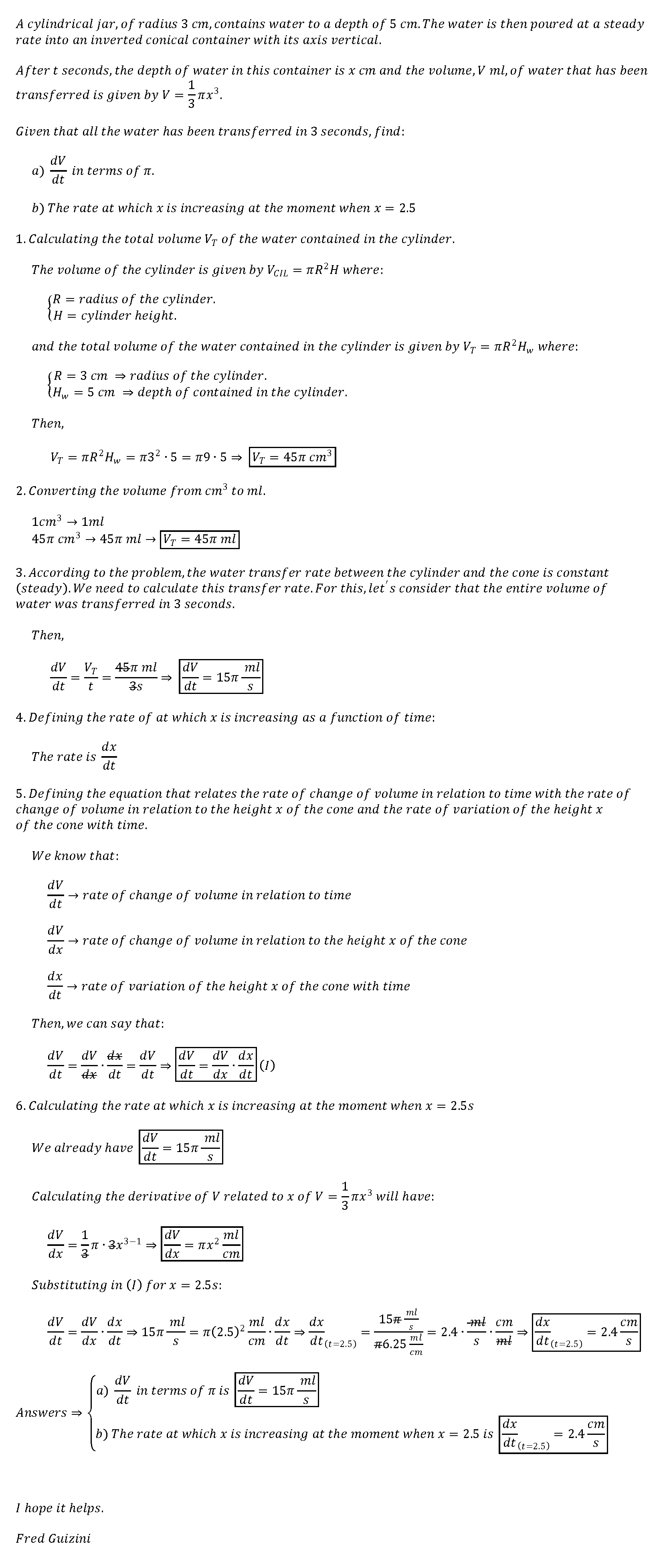
A cylindrical jar, of radius 3 cm, contains water to a depth of 5 cm. The water is then poured at a steady rate into an inverted conical container with its axis vertical. ?

After t seconds, the depth of water in this container is x cm and the volume,V ml, of water that has been transferred is given by #V=1/3pix^3#.
Given that all the water has been transferred in 3 seconds, Find

1) #(dV)/dt# in terms of #pi#
2) the rate at which x is increasing at the moment when x=2.5