A charged particle moves through a magnetic field perpendicular to its direction , then discuss about the kinetic energy and momentum of the particle whether they remain constant or change?

When a charge particle enters into a magnetic field of strength #B# with a velocity #v# at a perpendicular direction to that of #B#,we can say,Lorentz force acting is #vec F =q(vec v×vec B)#

So,F=qvB sin 90=qvB# where, #q# is its charge (taking magnitude of the vectors)

And this force acts in a direction perpendicular to both #B# and #v#

Now,suppose a case where this charged particle entered with velocity #v# directed along right,where #B# is directed into the plane of the page,so force acting on it will be upwards,so it will try to change the direction of its velocity to go up,but now force will act towards left,so it will try to go towrds left,so direction of velocity will change,and again force will act on it downwards and velocity will follow the change in direction,and this thing will continue,as a result the charge moves along a circular pathway with constant velocity,as no external force is acting along the direction of the velocity to change its magnitude,only direction changes.

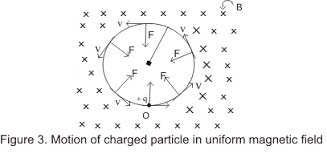
As a result,kinetic energy remains constant (#1/2mv^2#) and magnitude of momentum remains constant but direction changes along with the change in the direction of velocity.