A box with an initial speed of #3 m/s# is moving up a ramp. The ramp has a kinetic friction coefficient of #4/5 # and an incline of #( pi )/6 #. How far along the ramp will the box go?

This problem is most easily done by conservation of energy.
Three energy forms are involved:
A change in kinetic energy: #1/2mv_f^2-1/2mv_i^2#

A change in gravitational potential energy: #mgh#

Frictional heating: #muF_NDelta d#

Before we can continue, we need to be aware of a couple of complications

We must express #h# (the height the object rises) in terms of #DeltaD# (its displacement along the ramp).

#h=Deltadsin(pi/6)#

Also, we must note that the normal force on an incline is not equal to mg, but to #mgcostheta#, where #theta=pi/6# in this case.

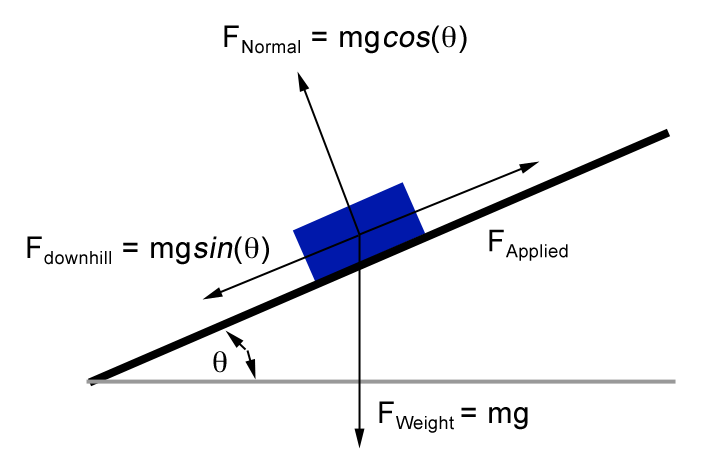
With all that looked after, our equation becomes

#1/2mv_f^2-1/2mv_i^2+mgDeltadsin(pi/6)+mumgcos(pi/6)Delta d=0#

Notice that we can divide every term by the mass m, (including the right side of the equation).

Also, you should check the equation for signs. The kinetic energy term will be negative - the box slows down. The potentail enrgy and frictional heat terms are both positive. Both of these energy forms increases.

Now since #v_f=0# , and inserting the values for #mu#, #v_i# and g, we get:

#-1/2(3)^2+(9.8)Deltad(0.5)+(4/5)(9.8)(0.866)Delta d=0#

#-4.5+4.9Deltad+6.79Deltad=0#

#11.79Deltad=4.5#

#Deltad=0.38m#