Question #aa923

For starters, you should know that the angular momentum quantum number, #l#, designates the energy subshell in which an electron is located inside an atom.

The magnetic quantum number, #m_l#, designates the specific orbital in which the electron is located.

As you know, orbitals are located in subshells, which in turn are located in energy shells.

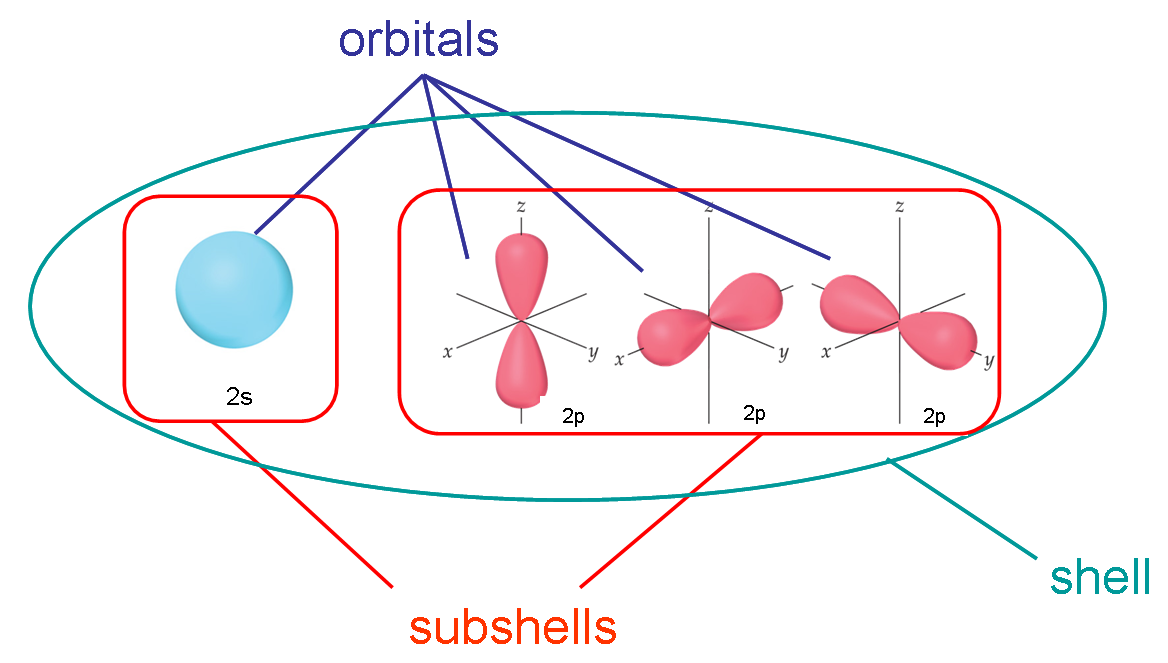
This implies that the values that the magnetic quantum number can take depend on the values of the angular momentum quantum number, which, of course, depend on the values of the principal quantum number, #n#, which designates the energy shell in which the electron is located.

Now, the number of orbitals located in a given subshell is given by

#color(blue)(ul(color(black)("no. of orbitals" = 2l + 1)))#

In other words, the number of values that the magnetic quantum number can take for a given subshell, i.e. for a given value of #l#, is given by #2l+1#.

Let's take the example illustrated in the image.

For the second energy level, #n=2#, you have two possible subshells, i.e. two values for #l#

• #l = 0 -># denotes the #2s# subshell
• #l=1 -># denotes the #2p# subshell

Now, the #2s# subshell can only hold #1# orbital because

#"no. of orbitals in 2s" = 2 * 0 + 1 = 1#

The #2p# subshell can hold #3# orbitals because

#"no. of orbitals in 2p" = 2 * 1 + 1 = 3#

In other words, the magnetic quantum number can only take #1# value for the #2s# subshell (or for any #s# subshell)

#n=2, l=0 implies m_l = 0#

and #3# values for the #2p# subshell (or for any #p# subshell)

#n=2, l=1 implies {(m_l = -1), (m_l = 0), (m_l = +1) :}#

You now know that #2l+1# will tell you the number of orbitals present in a given subshell.