Question #9f672

The thing to keep in mind here is that bismuth-203 undergoes beta positive decay, or positron emission, not beta minus decay, which more often than not is simply called beta decay.

https://en.wikipedia.org/wiki/Isotopes_of_bismuth

When a radioactive nuclide undergoes positron emission, a proton is converted to a neutron and a positron, #"e"^(+)#, the antiparticle of an electron, and an electron neutrino, #nu_"e"#, are emitted from the nucleus.

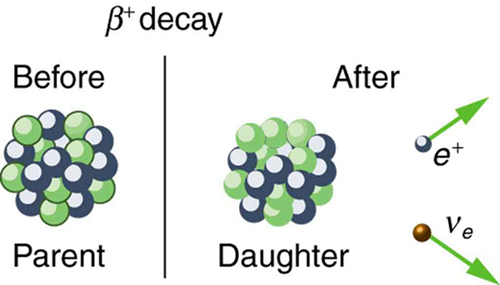
This means that--keep in mind that charge and mass are conserved in a nuclear reaction!

• the atomic number of the nuclide, #Z#, will decrease by #1#
• the mass number of the nuclide, #A#, will remain unchanged

So, you can write

#""_ (color(white)(1)83)^203"Bi" -> ""_ Z^A"?" + ""_ 1^0"e" + nu_ "e"#

The atomic number decreases by #1#, so

#83 = Z + 1 implies Z = 82#

The mass number remains unchanged, so

#203 = A + 0 implies A = 203#

Grab a Periodic Table and look for the element with the atomic number equal to #82#. You'll find that you're dealing with lead, #"Pb"#. This implies that the resulting isotope is lead_203.

The balanced nuclear equation that describes the position emission of bismuth-203 will thus look like this

#""_ (color(white)(1)83)^203"Bi" -> ""_ (color(white)(1)82)^203"Pb" + ""_ 1^0"e" + nu_ "e"#