Which of the following binary solutions of volatile liquids have negative deviation?

The mixture is #A)#, and has negative deviation, as indicated by #P_(AB)^"re" < P_(AB)^"id"#. This is because chloroform hydrogen-bonds with acetone, but before mixing, they had dipole-dipole interactions within themselves.

DISCLAIMER: LONG ANSWER!

Since the two liquids are volatile (i.e. they vaporize easily), we're going to have to find an expression for the total vapor pressure of the ideal binary mixture, #P_(AB)^"id"#. This will be compared with the real total vapor pressure, #P_(AB)^"re"#.

DETERMINING THE TOTAL VAPOR PRESSURE

We assume the vapor above the solution is ideal so that we can utilize Dalton's law of partial pressures:

#P_(AB)^"id" = P_A + P_B#

where #A# is the solvent and #B# is the solute. #""^"id"# indicates an ideal solution.

In an ideal solution, the solvent always follows Raoult's law, so we can write:

#P_A = chi_AP_A^@#

where the #@# indicates the pure component, and #chi# is the mol fraction of the component.

We assume the solution is also not dilute, since #chi_A = 0.4# is not a small mole fraction. Hence, the solute #B# also follows Raoult's law (rather than Henry's law), and we can write:

#P_B = chi_BP_B^@#

That means the vapor pressure of the mixture of volatile liquids is:

#P_(AB)^"id" = chi_AP_A^@ + chi_BP_B^@#

But since this is a binary mixture, i.e. two components, we know that the mol fractions add up to #1#, meaning #chi_A + chi_B = 1#, and thus:

#bb(P_(AB)^"id") = chi_AP_A^@ + (1 - chi_A)P_B^@#

#= bb(chi_A (P_A^@ - P_B^@) + P_B^@)#

The total vapor pressure assuming ideality is therefore:

#color(blue)(P_(AB)^"id") = 0.4("300 torr" - "800 torr") + "800 torr"#

#=# #color(blue)("600 torr")#

CLASSIFYING THE SOLUTION'S DEVIATION FROM IDEALITY

We're not done yet though. The real vapor pressure you've given us is #P_(AB)^"re" = "580 torr"#, which is less than what is predicted by Raoult's law.

That is indicative of negative deviation, which is depicted by this diagram:

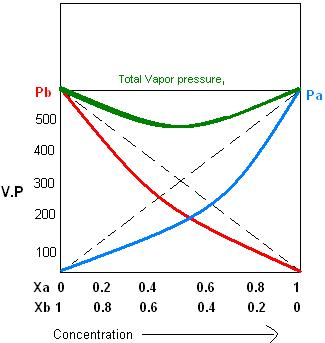
(The dashed diagonal lines and the horizontal line at the top are from Raoult's law, and the solid lines are the real vapor pressures.)

Negative deviation is when the average distance between solvent and solute particles is closer than in an ideal solution. This is when the energy of solute-solvent interaction (#AB#) is lower than the energy of solute-solute (#BB)# or solvent-solvent (#A A#) interaction.

This means that the intermolecular forces formed between solute and solvent are stronger than those that were present previously amongst the solute and solvent within themselves.

Recall the most important ones:

#"H"-"bonding" > "dipole-dipole" > "dispersion"#

CONSIDERING THE BINARY MIXTURE OPTIONS

We now examine the mixture choices.

#A)# chloroform with acetone

This is a tricky example, but chloroform has dipole-dipole interactions with itself, and acetone also has dipole-dipole interactions with itself... however, acetone hydrogen-bonds with chloroform!!

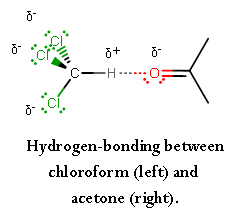
The three chlorine atoms are electron-withdrawing groups, which make the hydrogen very electropositive.

This is enough for the carbonyl oxygen on acetone to become a hydrogen-bond acceptor, polarizing the hydrogen atom and forming a hydrogen-bonding interaction.

Since hydrogen-bonding is stronger than dipole-dipole interactions on average, this mixture will have negative deviation, which agrees with how we got #P_(AB)^"re" < P_(AB)^"id"#. That is, the mixture is #A#.

#B)# chlorobenzene and bromobenzene

This mixture has two substances that only have dipole-dipole forces to exchange, and they therefore form essentially ideal solutions.

#C)# benzene with toluene

#D)# hexane with heptane

Both of these primarily have dispersion forces (toluene, #"C"_6"H"_5"CH"_3#, is mostly nonpolar), and it is not expected that any exchange of intermolecular forces leads to any more stabilizing or less stabilizing interactions. That is, these will be essentially ideal solutions.