Question #78ef0

1 Answer
A08
Apr 22, 2017

#6.36xx10^-11# units.

Explanation:

lessons.chemistnate.com

Body-Centered Cubic lattice structure is as shown in the figure. One atom is located at the lattice point at the center of the cube. Eight atoms are placed at eight lattice points located at the corners of the cube.

  • The atoms touch each other along the directions of body diagonals.
  • Only 1/8th of each atom in the corner belongs to the cell.
  • The one in the center of the cell entirely belongs to the unit cell.
  • As such there are 2 atoms per unit cell

Look at the geometry of the BCC structure as reproduced below:

chemteam.info

#d# is the length of the edge of a unit cell and
#4r# is the length of body diagonal.
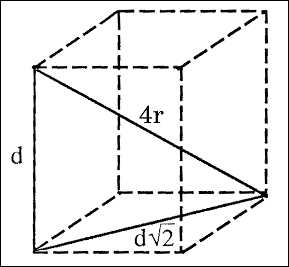
Where #r# is radius of atom forming the lattice structure.

From Pythagoras theorem #dsqrt2# is the length of any face diagonal
And

#(4r)^2=d^2 + (dsqrt2)^2#
#=>(4r)^2=3d^2 #
#=>r=sqrt3/4d #

Inserting given value in above we get
atomic radius of the element #r=sqrt3/4 1.469xx10^-10 #
#=>r=6.36xx10^-11# units.

Related questions
See all questions in Ionic Compounds
Impact of this question
2487 views around the world
You can reuse this answer
Creative Commons License