
Internal structure of a CRT is as shown in the figure above. It has five main parts listed below
- Electron gun.
- Deflection plate system.
- Fluorescent screen.
- Glass envelope.
- Base.
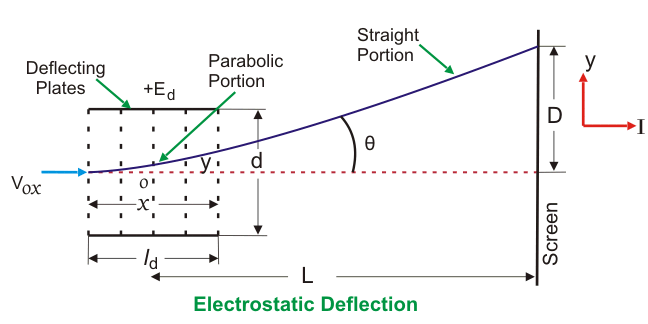
Electrostatic deflection sensitivity of CRT is associated with the deflection system as in the figure below.
A beam of electrons produced, focused and accelerated by the electron gun and its components enters the two deflecting plates with velocity #v_("o"x)#. A voltage of #E_d# is applied across the plates so that electric field produced is along #+y#-axis as shown.
If #V_a# is the voltage of the anode with respect to cathode, the kinetic energy gained by the electron of charge is #eV_a# which is also equal to #1/2mv_("o"x)^2#. Hence we hav the equation
#1/2mv_("o"x)^2=eV_a# ......(1)
Let #d# be distance between the plates. Electric field between the plates is given by #vecE=E_d/dhaty#.
Electrical Force on a electron of charge #e# is #vecF_y=eE_d/dhaty#
If #m# is the mass of charge then acceleration #a_y=eE_d/(dm)hat y#.
Writing kinematic equation for #y# deflection produced in time #t# and using the equation
#s=ut+1/2at^2# and inserting above values and noting that initial defection is #0# we get
#y=1/2(eE_d/(dm))t^2# ......(2)
As the velocity along the #x# direction is constant therefore we can write for displacement #x#
#x=v_("o"x)t# .....(3)
Inserting value of #t# from (3) in (2) we get
#y=1/2(eE_d/(dmv_("o"x)^2))x^2# .....(4)
This is an equation of parabolic path followed by electron in the deflection plate region. Just after leaving the deflection plates the electron beam travels in a straight line path and hits the screen at #y=L#.
If #l_d# is length of deflection plates slope of electron at the exit can be calculated by differentiating equation (4) and evaluating slope at the #x=l_d#. Which is
#(dy)/dx=(eE_d/(dmv_("o"x)^2))l_d#
Using equation (1) this can be rewritten as
#(dy)/dx=(E_dl_d)/(2dV_a)# .....(5)
For a small deflection in the deflecting plate region, #angletheta# can be approximated as shown in the figure. As such
#(dy)/dx=tantheta=D/L# .....(6)
where #L# is as shown in the figure. It is straight portion of the electron beam at the exit point extended backwards which meets the #x#-axis at the mid point of length #l_d#.
Using (5) and (6) we get
#D/L=(E_dl_d)/(2dV_a)#
#=>D/E_d=(Ll_d)/(2dV_a)#


