What is the change in internal energy of reaction when #"0.721 g"# of titanium is placed into a bomb calorimeter and allowed to combust, if the temperature rises from #25^@ "C"# to #53.8^@ "C"#? #C_"cal" = "9.84 kJ/K"#.

I'm getting #D#.

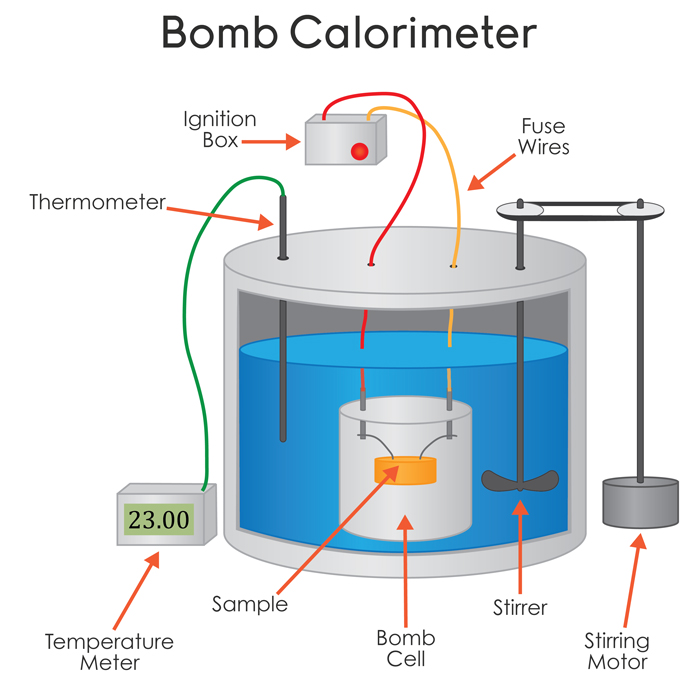
A bomb calorimeter by definition is a constant-volume calorimeter. These are rigid and closed, generally with a large water bath to insulate the system.

This is in such a way that heat generated or absorbed due to the reaction is equal to the heat absorbed or generated (respectively) by the calorimeter (conservation of energy).

That means you must recall that #DeltaE = q_V#, where #q_V# is the heat flow #q# at constant volume, since #DeltaE = q + w = q - cancel(PDeltaV)^(0)#.

The following data was given:

• #m_"Ti" = "0.721 g"#

• #DeltaT = T_2 - T_1 = 53.8^@ "C" - 25^@ "C" = 28.8^@ "C"#

• #C_"cal" = "9.84 kJ/K"#, or #"9.84 kJ/"^@ "C"#
  (note: #DeltaT# in #""^@ "C"# is the same as #DeltaT# in #"K"#, but the individual temperature values are NOT.)

Since you were given the heat capacity of the calorimeter, you can calculate #q_V# for the calorimeter itself:

#color(green)(q_(V,"cal")) = m_"cal" c_"cal"DeltaT#

#= C_"cal"DeltaT#

#= ("9.84 kJ/"^@ "C")(28.8^@ "C")#

#=# #color(green)("283.4 kJ")#

where #c# is the specific heat capacity in #"kJ/mol"^@ "C"# and #C# is the heat capacity in #"kJ/"^@ "C"#. Do not confuse them---they are not the same!

So, we can set this equal to #DeltaE_"cal"# as we stated earlier:

#DeltaE_"cal" = q_(V,"cal") = "283.4 kJ"#

Finally, in #"kJ/mol Ti"#, we must calculate the #"mol"#s of #"Ti"# combusted:

#n_"Ti" = m_"Ti"/(M_(m,"Ti"))#

#= "0.721 g" xx "1 mol Ti"/"47.867 g" = "0.0151 mols Ti"#

In the end, calculating the change in internal energy #DeltaE# (some might call it #DeltaU# to not confuse it with the total energy) for the reaction, which is also the heat of reaction in this case, would ask you to realize that:

#q_(V,"rxn") = -q_(V,"cal") = -"283.4 kJ"#

when energy is conserved. Therefore, for the reaction, we have that:

#color(blue)(DeltaE_"rxn") = q_"rxn"/(n_"Ti")#

#= -"283.4 kJ"/("0.0151 mols Ti")#

#= -"18814.3 kJ/mol"#

#= color(blue)(-1.89 xx 10^4)# #color(blue)("kJ/mol")#