How does the atomic radius of argon compare to that of chlorine?
2 Answers
I see many images online where argon's atomic radius is smaller than that of chlorine. Here are a few diverse ones:
Calculated Atomic Radii (pm)
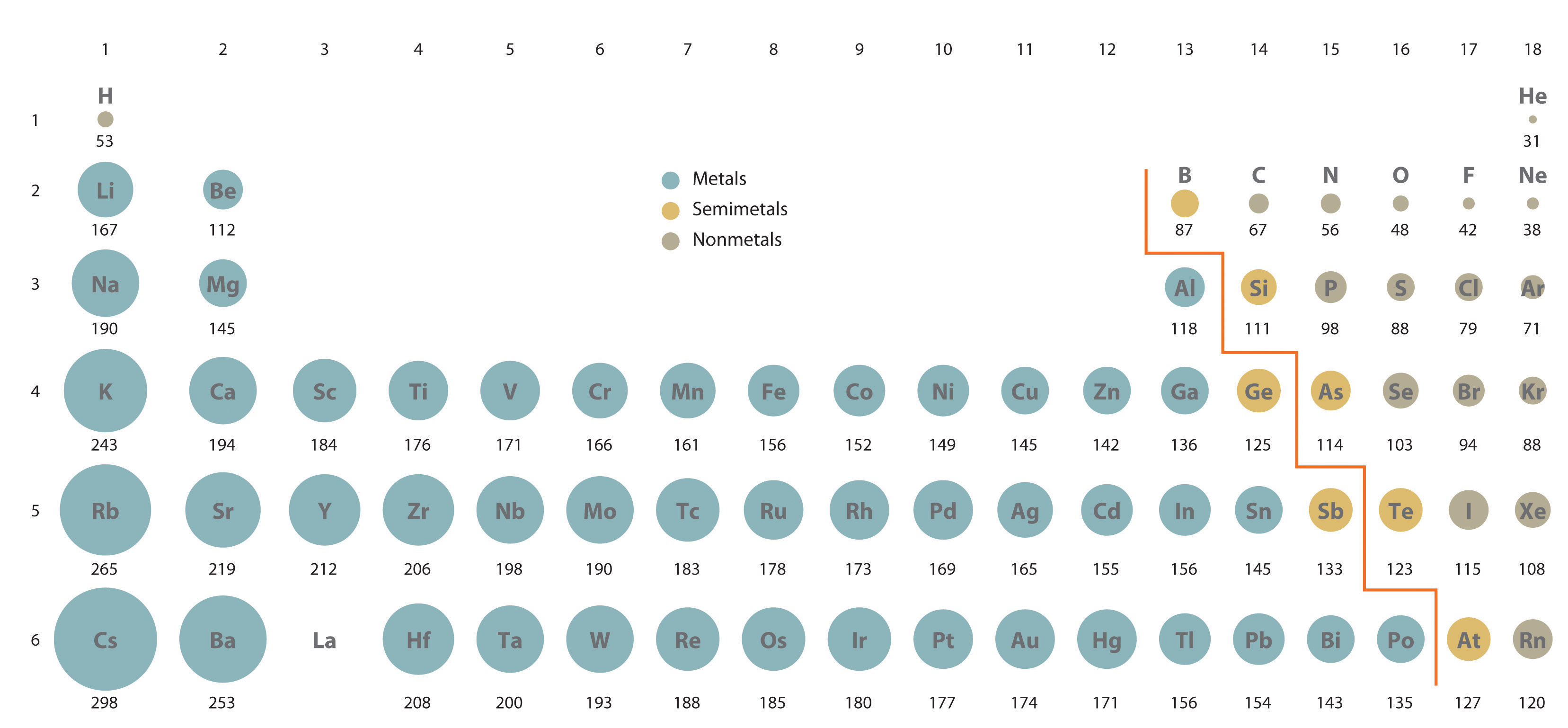
Calculated Atomic Radii (pm)

Covalent vs. Atomic Radius (pm)
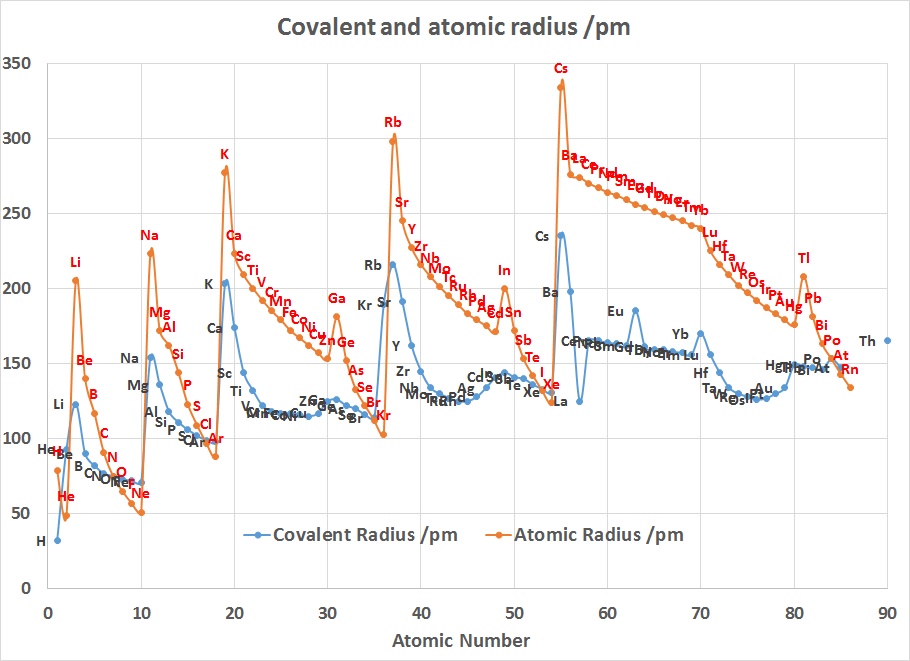
Generally the argument is the increased effective nuclear charge
Using Slater's Rules, we could calculate
#color(blue)(Z_(eff) = Z - S)# where:
#Z# is the atomic number.#S# is the shielding constant, dependent on the orbital chosen.
To determine the shielding constant, some relevant parts of the rules are (Inorganic Chemistry, Miessler et al., pp. 30, 32):
- Group the atomic orbitals as
#(1s)(2s,2p)(3s,3p)(3d)(4s,4p)(4d)(4f)(5s,5p)(5d)# (and so on) - Electrons in groups to the right do not shield electrons in groups to the left.
- For a particular chosen ns or np valence electron:
- Each other electron in the same group shields it, and contributes
#0.35# to the value of#S# . - An exception is that 1s electrons contribute
#0.30# instead of#0.35# if in the same group. - Each electron in
#n-1# groups contribute#0.85# to#S# . - Each electron in
#n-2# or lower groups contributes#1.00# to#S# .
- Each other electron in the same group shields it, and contributes
So, for one of chlorine's
#1s^2 2s^2 2p^6 3s^2 3p^5#
#S_(3p) = stackrel("1s")(overbrace(2xx1.00)) + stackrel("2s")(overbrace(2xx0.85)) + stackrel("2p")(overbrace(6xx0.85)) + stackrel("3s")(overbrace(2xx0.35)) + stackrel("3p")(overbrace(4xx0.35))#
#= 10.9#
#S_(3s) = stackrel("1s")(overbrace(2xx1.00)) + stackrel("2s")(overbrace(2xx0.85)) + stackrel("2p")(overbrace(6xx0.85)) + stackrel("3s")(overbrace(1xx0.35)) + stackrel("3p")(overbrace(5xx0.35))#
#= 10.9#
#color(blue)(Z_(eff,"Cl")^(3p) = Z_(eff,"Cl")^(3s)) = 17 - 10.9 = color(blue)(6.1)#
On the other hand, for one of argon's
#1s^2 2s^2 2p^6 3s^2 3p^6#
#S_(3p) = stackrel("1s")(overbrace(2xx1.00)) + stackrel("2s")(overbrace(2xx0.85)) + stackrel("2p")(overbrace(6xx0.85)) + stackrel("3s")(overbrace(2xx0.35)) + stackrel("3p")(overbrace(5xx0.35))#
#= 11.25#
#color(blue)(Z_(eff,"Ar")^(3p) = Z_(eff,"Ar")^(3s)) = 18 - 11.25 = color(blue)(6.75)#
Therefore, we would expect that argon's valence electrons are more attracted to the nucleus, and so it should have a smaller radius.
Actually, Cl has a greater atomic radius than Ar because of the greater attraction from the nucleus in Ar.
Explanation:
Relative atomic radius is mostly determined by how many electron shells there are and by the number of protons that are in the nucleus.
When going doing the groups (the columns) of the periodic table, the atomic radius will increase because the element below will have one more energy level more than the element above it. That level will have a higher energy and be farther away from the nucleus. Imagine Bohr's model of an atom. The nucleus is a dot in the middle, and the energy levels are circles of increasing radii.
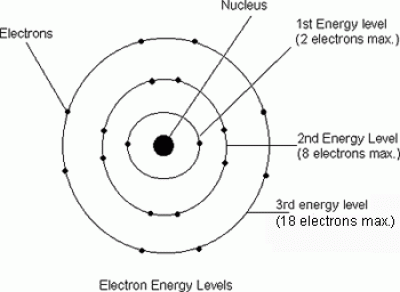
By adding another energy level, you draw a larger circle around the other circles, effectively increasing the radius of that atom.
(Note: Bohr's model has been proven to be inaccurate, but the concept is still relevant.)
When going left to right on the periodic table, the atomic radius will decrease. This happens because no shells are being added since all the electrons in that period (the row) belong in the same shell. When you go left to right, you add an electron and proton. Because the proton has a higher attraction than the electron, the proton will win over the electron-electron repulsion between the outer electron shell and other shells and essential shrink the outer electron shell. This will mean that adding a proton and electron (or going right in a period) will decrease the atomic radius.
Because Argon is to the right of Chlorine and on the same period, Argon has an atomic radius less than Chlorine's atomic radius.
(Picture Credit: Dublin Schools)


