What are the quantum numbers necessary to specify a #5p# subshell?

As you know, quantum numbers are sused to describe the exact location and spin an electron can have in an atom.

A total of #4# quantum numbers are used for this purpose, with every electron that's part of a given atom having a unique set of quantum numbers associated with its position ans spin.

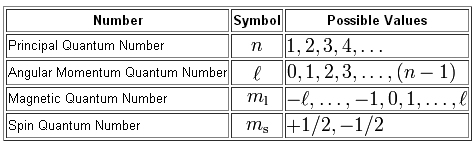
Now, the principal equantum number, #n#, gives you the energy level on which you can find an electron.

Each energy level contains a specific number of subshells, given the possible values the angular momentum quantum number, #l#, can take.

For example, the second energy level is characterized by #n=2#. As you can see in the table, #l# can take values rnging from #0# to #n-1#.

This means that the second energy level will have a total of #2# subshells.

In your case, the energy level is given by the number that's placed in front of the letter p, which denotes a specific subshell.

So, the principal quantum number, #n#, for the 5p-subshell is #color(green)(n=5)#.

Now, the any p-subshell is characterized by #l=1#. Similarly, any s-subshell is characterized by #l=0#, any d-subshell by #l=2#, and so on.

Therefore, the value of angula momentum quantum number will be #color(green)(l=1)#.