A sample of a compound containing the elements silver, carbon, and oxygen, has a mass of #"1.372 g"#. What are the empirical and molecular formulas?

So, you know that you're dealing with a compound that contains carbon, oxygen, and silver. Moreover, you're using a sample that has a total mass of #"1.372 g"#.

Since they tell you that this sample was found to contain #"0.288 g"# of oxygen and #"0.974 g"# of silver, you can use the total mass of the sample to determine how much carbon it contains.

#m_"sample" = m_"oxygen" + m_"carbon" + m_"silver"#

#m_"carbon" = "1.372 g" - ("0.288 g" + "0.974 g")#

#m_"carbon" = "0.110 g"#

Now, in order to determine what the empirical formula of the compound is, you need to know two things

• how many moles of each element you get in that sample
• what the mole ratio between the elements that make up the compound is

To get the number of moles of each element, use its mass and known molar mass, which tells you exactly what the mass of one mole of said element is.

#0.110color(red)(cancel(color(black)("g C"))) * "1 mole C"/(12.011color(red)(cancel(color(black)("g C")))) = "0.009158 moles C"#

#0.288color(red)(cancel(color(black)("g O"))) * "1 mole O"/(15.9994color(red)(cancel(color(black)("g O")))) = "0.01800 moles O"#

#0.974color(red)(cancel(color(black)("g Ag"))) * "1 mole Ag"/(107.87color(red)(cancel(color(black)("g Ag")))) = "0.009029 moles Ag"#

Now, to get the mole ratios that exist between these elements, divide these values by the smallest one. This will get you

#"For C: " (0.009158color(red)(cancel(color(black)("moles"))))/(0.009029color(red)(cancel(color(black)("moles")))) = 1.0143 ~~ 1#

#"For O: " (0.01800color(red)(cancel(color(black)("moles"))))/(0.009029color(red)(cancel(color(black)("moles")))) = 1.994 ~~ 2#

#"For Ag: " (0.0009029color(red)(cancel(color(black)("moles"))))/(0.009029color(red)(cancel(color(black)("moles")))) = 1#

This means that the empirical formula of the compound, which tells you what the smallest whole number ratio between the atoms that make up the compound is, will be

#"Ag"_1"C"_1"O"_2 implies "AgCO"_2#

To get the molecular formula, which tells the exact ratio that exists between the atoms that make up a compound, you need to use the know molar mass of the compound.

More specifically, you can think of the empirical formula as being a building block for the actual chemical formula. Your job now is to determine how many building blocks would be needed to make the actual compound.

To do that, find the molar mass of the empirical formula by adding the molar mass of every atom it contains

#(107.87 + 12.011 + 15.9994 xx 2)color(red)(cancel(color(black)("g/mol"))) * color(blue)(n) = 303.8color(red)(cancel(color(black)("g/mol")))#

#1512.88 * color(blue)(n) = 303.8#

So, how many building blocks would you need to get the compound?

#color(blue)(n) = 303.8/151.88 = 2.0003 ~~ 2#

Therefore, the molecular formula for your compound is

#("AgCO"_2)_color(blue)(2) = "Ag"_2"C"_2"O"_4 -># silver oxalate

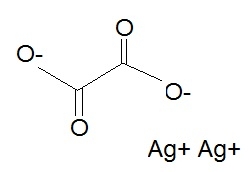
The empirical formula of a compound represents the lowest whole number ratio of elements in the compound.

We are given the total mass of a sample, and the mass of oxygen and silver, but not carbon. We need to calculate the mass of carbon by subtracting the sum of oxygen and silver from the total mass.

#1.372"g"-(0.288"g O"-0.974"g Ag")="0.110 g C"#

Determine Moles of Each Element
Divide the mass of each element by its molar mass (atomic weight on the periodic table in grams/mole, or g/mol).

#"C":##0.110cancel"g C"xx(1"mol C")/(12.0107cancel"g C")="0.00915 mol C"#

#"O":##0.288cancel"g O"xx(1"mol O")/(15.999cancel"g O")="0.0180 mol O"#

#"Ag":##0.974cancel"g Ag"xx(1"mol Ag")/(107.8682cancel"g Ag")="0.00903 mol Ag"#

Determine Mole Ratios
Divide the moles of each element by the lowest number of moles.

#"C":##(0.00915 cancel"mol")/(0.00903 cancel"mol")=1.01~~1"#

#"O":##(0.0180cancel"mol")/(0.00903cancel"mol")=1.99~~2"#

#"Ag":##(0.00903cancel"mol")/(0.00903cancel"mol")=1.00#

The empirical formula is #"AgCO"_2#.

Determine the Empirical Formula Mass

#(1xx107.8682"g/mol Ag")+(1xx12.0107"g/mol C")+(2xx15.999"g/mol O")="151.9 g AgCO"_2#

Determine Molecular Formula

Divide the molecular molar mass of the compound by the empirical formula mass.

#(303.8cancel"g")/(151.9cancel"g")=2#

Multiply the empirical formula times #2# to get the molecular formula.

The molecular formula is #"Ag"_2"C"_2"O"_4"#.