Question #a288d

The idea behind this problem is that Earth's roration produces a centripetal acceleration, which is directed towards the center of rotation, that reduces the gravitational acceleration, #g#.

This centripetal acceleration is defined by the equation

#a = omega * r^2#, where

#omega# - the angular velocity of the Earth;
#r# - the radius of the Earth.

The force that creates this centripetal acceleration is called the centripetal force and is pointed towards the center of ration as well.

#F_"centripetal" = m * a = m * omega * r^2#

Now, an object that is in a rotating reference frame, i.e. a reference frame that is rotating with the system, will be acted upon by the centrifugal force.

This "force" (it is not a force per se) points outwards from the center of rotation and actually replaces the centripetal force.

#F_"centrifugal" = m * omega * r^2#

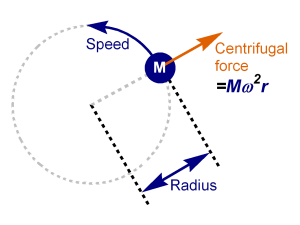
This means that, for a person that's rotating at the equator, this centifugal force will actually oppose gravity.

This means that the weight of the person at the equator will actually be

#cancel(m) * g_(eq) = cancel(m) * g - cancel(m) * omega * r^2#

For future reference, #g_(eq)# is actually called the acceleration due to gravity at the equator.

As you can see, the magnitude of #g_(eq)# depends on #omega#, which is the angular speed of rotation.

This bigger #omega# gets, the smaller #g_(eq)# will become, since you're subtrating an increasingly bigger number, #omega * r^2#, from #g#.

As a conclusion, the faster the Earth spins, the lower #g_(eq)# will be, which in turn means that a person's weight decreases.