What is the standard form of the parabola with a vertex at (5,5) and a focus at (5,-7)?

#"Since the vertex and focus both lie on the principal axis"#

#"and both have an x-coordinate of 5 with the focus below"#
#"the vertex then this is a vertically opening down parabola"#
#"with equation"#

#•color(white)(x)(x-h)^2=4a(y-k)#

#"where "(h,k)" are the coordinates of the vertex and a is"#
#"the distance from the vertex to the focus"#

#(5,5)to(5,-7)rArra=-12#

#"here "(h,k)=(5,5)#

#(x-5)^2=-48(y-5)#

#" "#
We are given the Vertex of a parabola and the Focus.

Vertex is a at #color(red)((5,5)#

Focus is a at #color(red)((5,-7)#

A Parabola is the set of #color(red)((x,y)# ordered pairs that are equal distance from its Focus and its Directrix.

Hence, the directrix must be in the opposite direction from the focus.

To start off, we plot these points on a graph:

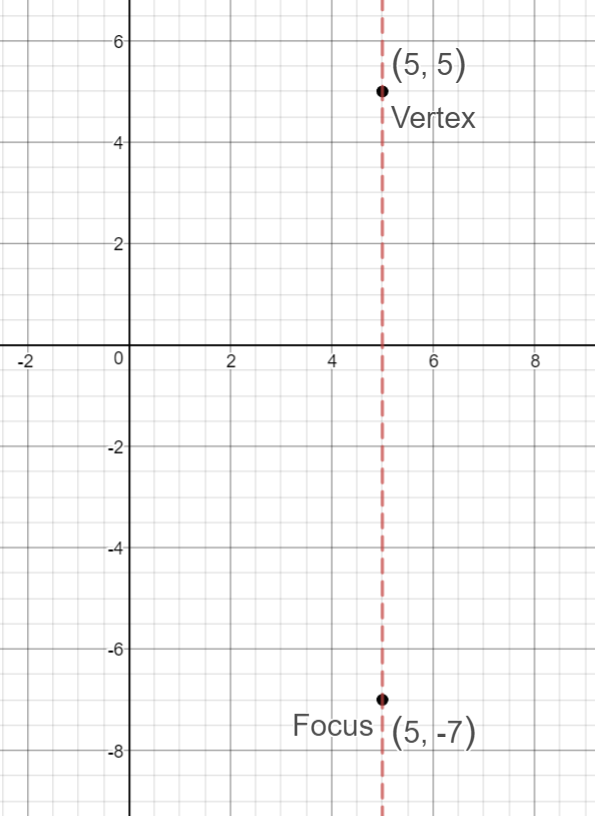
From the image above, we can understand that the focus lies below the x-axis and hence the directrix must lie above the x-axis.

The parabola will have the focus inside the curve (wraps around its focus).

So, the parabola will open downward containing the focus.

The equation of the parabola is:

#color(red)((x-h)^2=4p(y-k)#, where

#color(red)((h,k)# is the vertex

In the equation, if the p-value is negative, the parabola opens in the negative direction, like in this problem.

p-value refers to the distance between the vertex and the focus.

We can see that the value of #color(red)(p = (-12)#

We have the values of #color(red)(x, h and p#

Let us now get the equation of the general form of the parabola using the following formula:

#color(red)((x-h)^2=4p(y-k)#

#(x-5)^2=4(-12)(y-5)#

#x^2-10x+25=(-48)(y-5)#

#x^2-10x+25=-48y+240#

Subtract #color(red)((240)# from both sides:

#x^2-10x+25-240=-48y#

#-48y=x^2-10x-215#

Mulitply by #color(red)((-1)# both sides

#48y=-x^2+10x+215#

#color(blue)( :. y= (1/48)(-x^2+10x+215)#

This is the equation of the parabola we must find

Now we will examine the graph below to and verify all the intermediate results we found earlier:

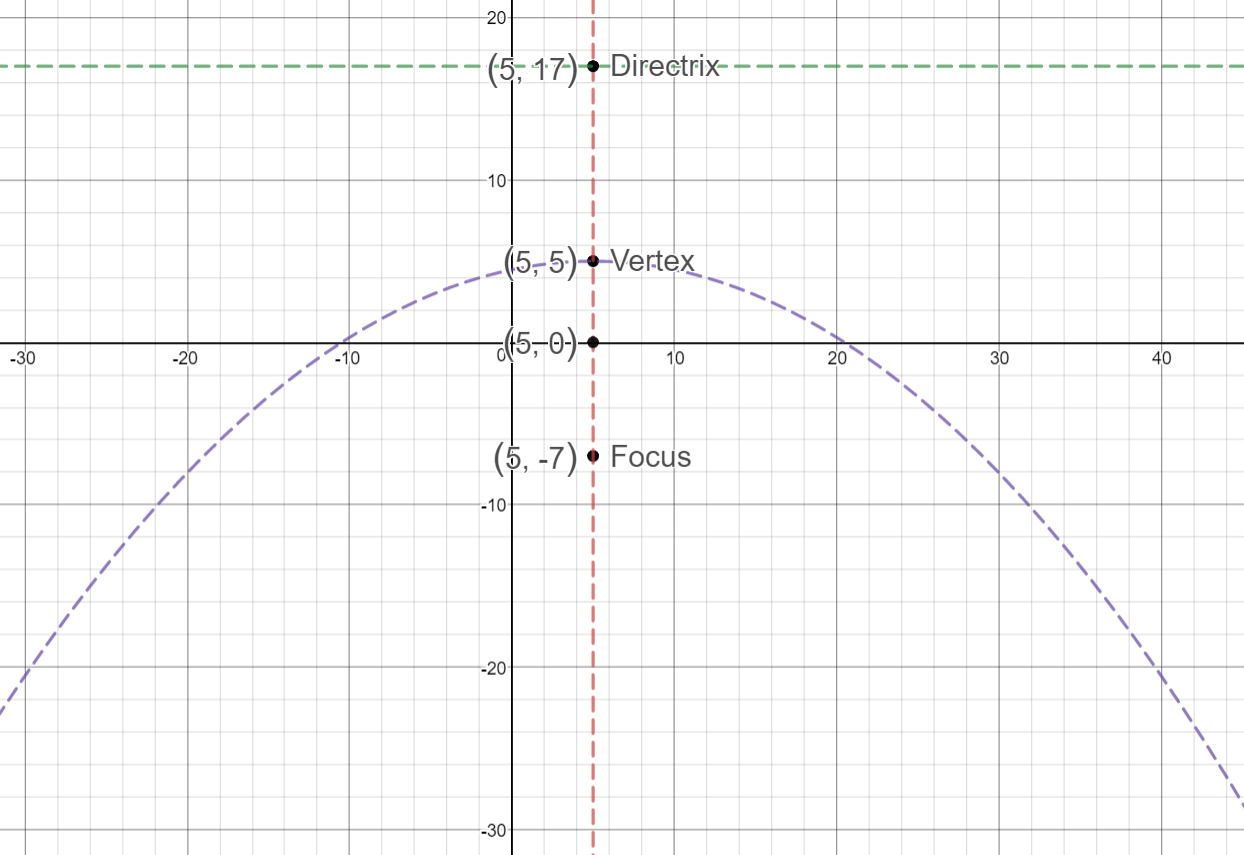
I hope this helps.