250 ml of oxygen gas is collected over water at a pressure of 1.27 atm and a temperature of 16 degrees C. What volume of dry gas will there be at 105 kpa and -22 degrees C?

We can gather the following information from the first sentence:

#V_1 = "250 mL"#
#P = "1.27 atm"# (this is not #P_1#; we'll find# P_1# using this value)
#T_1 = 16 " degrees C"#

Here's what we have from the second sentence:

#V_2 = "that's what we need to find"#
#P_2 = "105 kPa"#
#T_2 = -22 " degrees C"#

Let's handle the unit conversion first. Since the temperature values are given in degree C, we'll need to convert it to Kelvin for use in any Gas Laws. Here's how we do it:

#T_1 = 16 " degrees C" + 273 = 289 K#
#T_2 = -22 " degrees C" + 273 = 251 K#

Looks like the pressures are given in 2 different units. Let's convert #P_2# to atm (no particular reason, you can convert to any pressure unit as long as both pressures are in the same unit). [1 atm = 101.325 kPa].
#P_2 = "105 kPa"*(1 " atm")/(101.325 " kPa")=1.04 " atm"#

As mentioned earlier, the pressure given in the first sentence is not #P_1#. This is because 1.27 atm is the pressure of oxygen gas and water vapor at 16 degrees C. Therefore, we'll use Dalton's Law of Partial Pressures to find the pressure of oxygen (#P_1#):

#P_"total"= P_"Oxygen" + P_"water"#

Looks like #P_"water" = 13.6 " torr"# at 16 degrees C from the table:

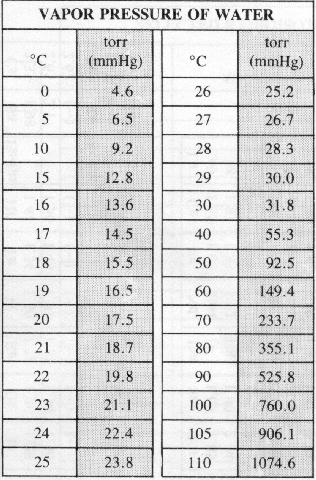
Let's convert that to atm. [1 atm = 760 torr]
#P_"water" = 13.6 " torr"*(1 " atm")/("760 torr")=0.0179" atm"#

Plugging in to the Dalton's Law of Partial Pressures,

#P_"total"= P_"Oxygen" + P_"water"#
#1.27" atm" = P_1+0.0179 " atm"#
#P_1=1.27 - 0.0179 = 1.25" atm"#

Let's update all the values we have so far:

#V_1 = "250 mL"#
#P_1=1.25" atm"#
#T_1 = 289 K#

#V_2 = "that's what we need to find"#
#P_2 = 1.04 " atm"#
#T_2 = 251 K#

Rearranging Combined Gas Law to find #V_2#:
#(P_1*V_1)/T_1=(P_2*V_2)/T_2#

#V_2=(P_1*V_1*T_2)/(T_1*P_2)=(1.25 atm*250 mL*251K)/(289K*1.04atm)=261 mL#