If f is a continuous function such that #int_0^xf(t)dt=xe^(2x)#+ #int_0^xe^-tf(t)dt# for all x, find an explicit formula for f(x) ?
I'm not sure how to use the fundamental theorem of calculus to solve for this problem.
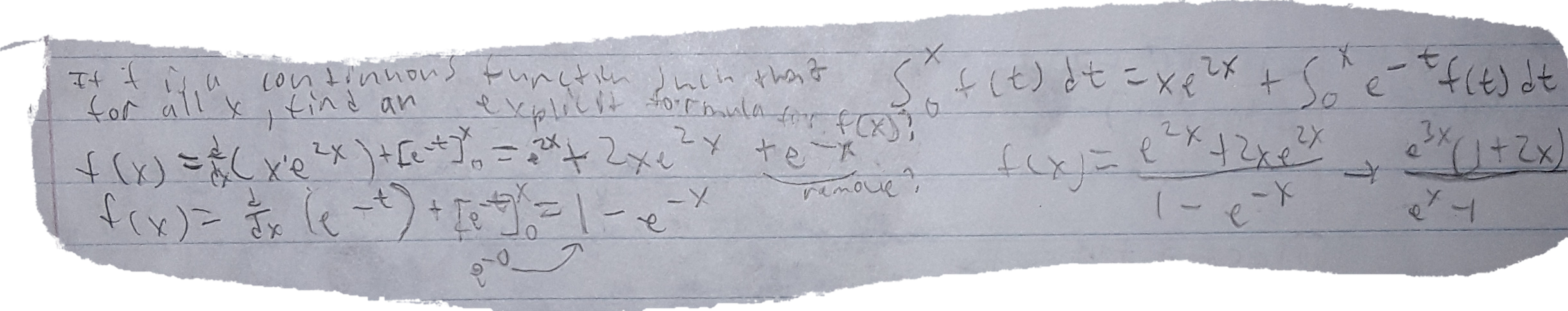
I don't think I wrote the f(x)=? in the correct formal form.
I do know that you first take the derivative of the function and add and plug in the values from the bounds of the function?
Also I am unsure of what to do with the "#+e^-x# " in the first part?
Thanks so much for your help in advance
I'm not sure how to use the fundamental theorem of calculus to solve for this problem.
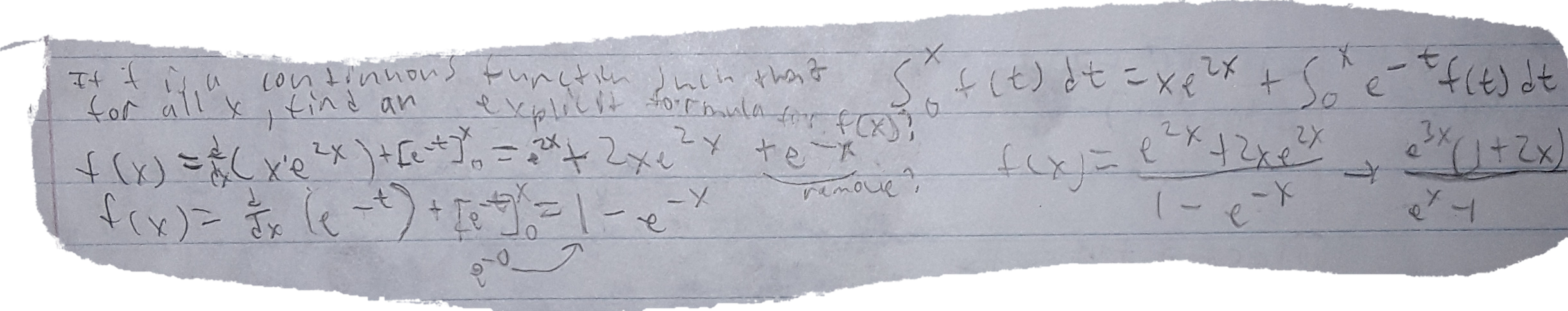
I don't think I wrote the f(x)=? in the correct formal form.
I do know that you first take the derivative of the function and add and plug in the values from the bounds of the function?
Also I am unsure of what to do with the "
Thanks so much for your help in advance
1 Answer
# f(x) = ((2x + 1)e^(2x))/(1 - e^(-x)) #
Explanation:
We have:
# int_0^x \ f(t) \ dt=xe^(2x) + int_0^x \ e^(-t)f(t) \ dt #
If we differentiate wrt
# d/dx \ int_0^x \ f(t) \ dt = d/dx \ xe^(2x) + d/dx \ int_0^x \ e^(-t)f(t) \ dt #
The Fundamental Theorem of Calculus tell us that:
# d/dx \ int_0^x \ f(t) \ dt = f(x) #
Thus if we apply this theorem, we have:
# f(x) = d/dx \ xe^(2x) + e^(-x)f(x) #
And now we can apply the product rule:
# f(x) = (x) (d/dx e^(2x)) + (d/dx x)(e^(2x)) + e^(-x)f(x) #
# \ \ \ \ \ \ \ = (x) (2e^(2x)) + (1)(e^(2x)) + e^(-x)f(x) #
# \ \ \ \ \ \ \ = 2xe^(2x) + e^(2x) + e^(-x)f(x) #
# :. f(x) - e^(-x)f(x) = 2xe^(2x) + e^(2x) #
# :. (1 - e^(-x))f(x) = (2x + 1)e^(2x) #
# :. f(x) = ((2x + 1)e^(2x))/(1 - e^(-x)) #

