Can someone help me answer this question? 'find the exact value of Cos theta, given sin theta = 5/9 and cos theta < 0

3 Answers
Gió
Jun 27, 2018

I got #0.831#

Explanation:

Have a look:
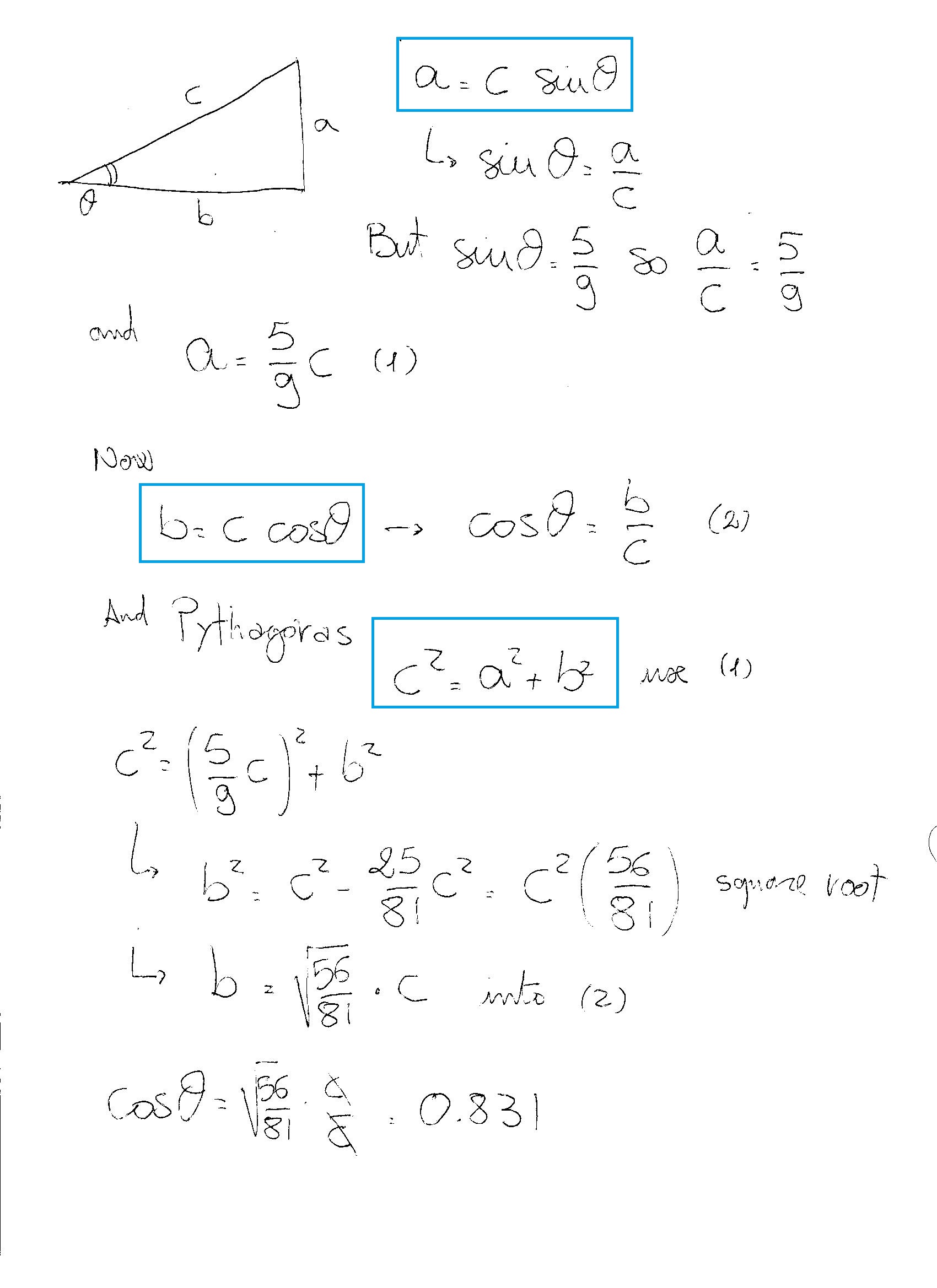
enter image source here

PJ
Jun 27, 2018

#cos(theta)= -0.83147941928309808568527749607034#

= -0.8315 (4dp)

Explanation:

Find #theta# (Note: there are 2 possible angles for #sin(theta) = 5/9 #)
#Sin^-1(5/9) = 33.748988595888587817404332744458 (degrees)#

The sin will also have the value #(5/9)# at

#180-33.748988595888587817404332744458#

#=146.25101140411141218259566725554 (degrees)#

If you are not sure which of these angles will have cos < 1, try both.
(note that the numbers for both angles are actually the same but one is positive and the other is negative)

#cos(33.748988595888587817404332744458)#
#=0.83147941928309808568527749607034#

#cos(146.25101140411141218259566725554)#
#=-0.83147941928309808568527749607034#

(so #theta# is the 146.25101.... value)

Jim G.
Jun 27, 2018

#costheta=-sqrt56/9#

Explanation:

#"using the "color(blue)"trigonometric identity"#

#•color(white)(x)sin^2theta+cos^2theta=1#

#rArrcostheta=+-sqrt(1-sin^2theta)#

#"given "sintheta=5/9" and "costheta<0#

#"then "theta" is in the second quadrant"#

#costheta=-sqrt(1-(5/9)^2)#

#color(white)(costheta)=-sqrt(1-25/81)#

#color(white)(costheta)=-sqrt(56/81)=-sqrt56/9#

Related questions
Impact of this question
7429 views around the world
You can reuse this answer
Creative Commons License