Sales in a fast food restaurant is believed to follow a normal distribution with mean of 12 and variance of 5?. Find the probability that the sales?.a)between 10 and 15, b)is less than 8 ,c) more than 11

You will need your formula booklet / data sheet / similar in order to answer this.

Start out my drawing a sketch of the normal distribution (a bell curve symmetrical about the mean). Label the mean value at the middle of the curve on your x axis.

Then, label the limits that are stated in the question (I will work through question 1 as an example, but this method is identical for each part); so you should have a point either side of your mean (as 10 < 12 < 15) as below:

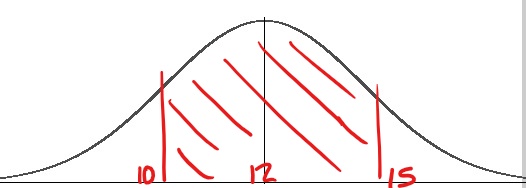
So now you need to find that middle highlighted area (as the area under a normal distribution = the probability of events in that range occuring). You do this using the normal distribution tables in your formula booklet / data sheet.

To determine the area, you actually have to work out two areas. This is because you can only work out the area from '0' (the far left) up to one limit at a time. So, first you need to work out the area up to 15 (shown below):

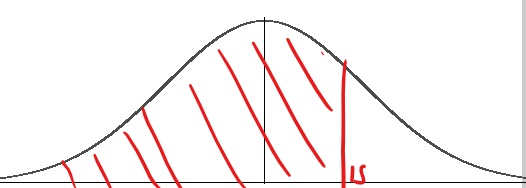
To find the area (the probability) up to that point, you need to convert the limit in to a 'z' value. This is done by the calculation:

#z = (x - mu)# / #sigma#
= (15 - 12) / #sqrt5#
= 1.34 (two decimal places)

Then all you need to do is look up that z value in your normal distribution table to find the probability:

P = 0.90988

Now repeat the whole process for the other limit (10). This time, you will get a negative z value as shown below:

#z = (x - mu)# / #sigma#
= (10 - 12) / #sqrt5#
= -0.89 (two decimal places)

When you look this up in your tables, the area that you are being given is actually this (shown below):

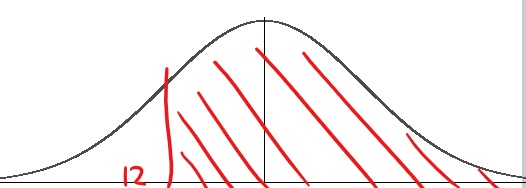
We don't want this area. We actually want to find that white bit that isn't highlighted - this is because when we take this white bit from the area up to 15, we will get our original highlighted section. So to find this white area, just subtract the area given by the tables from 1 (as total area = total probability = 1):

P = 1 - 0.81327 = 0.18673

And finally, to find the original area:

P (10 < X < 15) = 0.90988 - 0.18673
= 0.72315
= 0.723 (3 significant figures)