How do you subtract #(w ^ { 2} + 4w + 4) - ( w + 2)#?

Question

1783 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-05-31T14:08:22

May 31, 2018

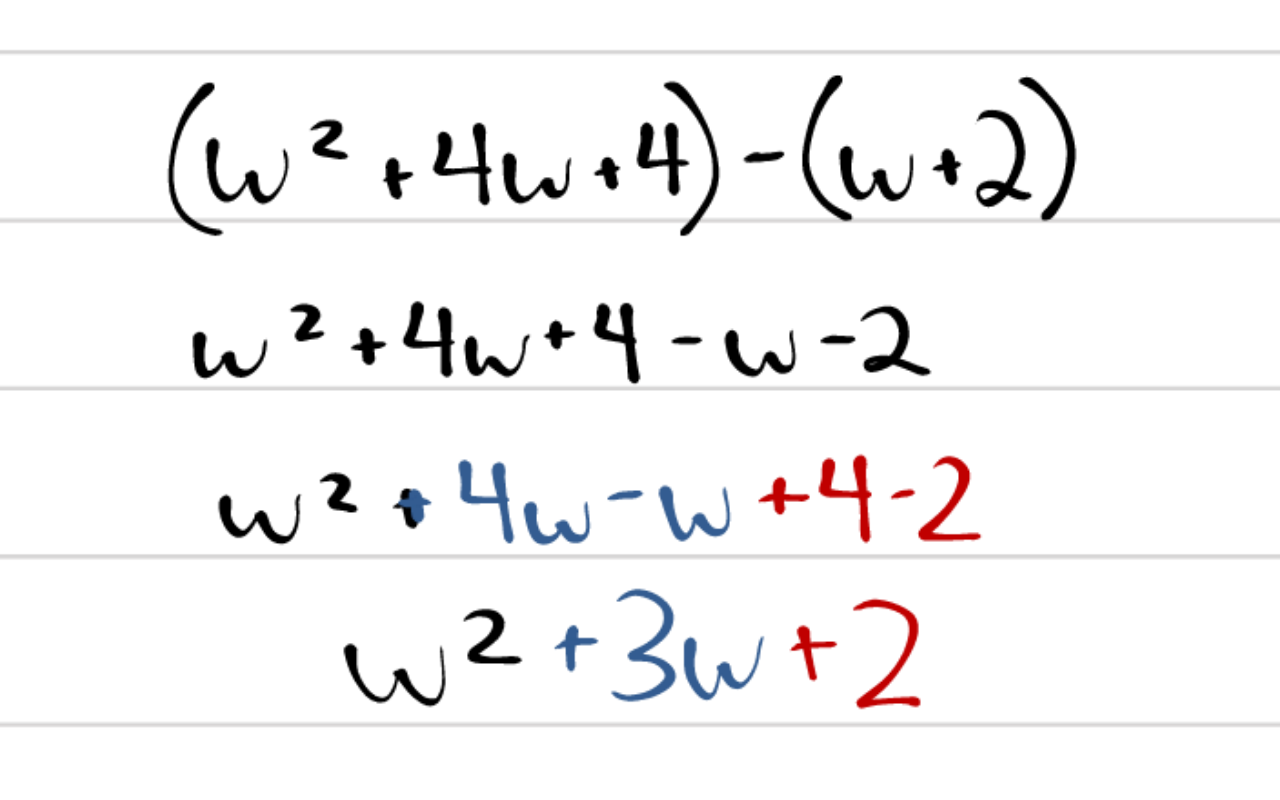
www.nosource.com

Answer 2 · 2018-05-31T14:11:01

#omega = -2# and #-1#

As per the question, we have

#(omega^2 +4omega + 4) - (omega + 2)#

And I assume that this is a solvable quadratic equation, #f(omega) = 0#

#:.(omega^2 +4omega + 4) - (omega + 2) = 0#

Opening the brackets, we get

#omega^2 +4omega + 4 - omega - 2 = 0#

Now as per our Pre-Algebra knowledge

#omega^2 + 3omega + 2 = 0#

#:.(omega + 1)(omega + 2) = 0#

#:. omega = -1, -2#

Hence, the answer.

Answer 3 · 2018-05-31T14:19:15

#w^2+3w+2#

The key here is that we subtract terms with the same degree from each other.

Since there is no other #w^2# term, we will just be subtracting

#color(blue)(4w-w)#

and

#color(cyan)(4-2)#

Putting it all together, we get

#w^2+color(blue)(4w-w)+color(cyan)(4-2)#

which simplifies to

#w^2+3w+2#

Hope this helps!