How do I Find P ( 2 < X < 7 )? (Stats)

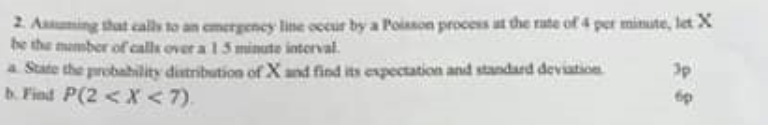
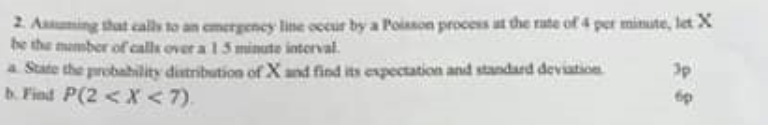
I Got the part A, but don't get how to do part B.enter image source here

1 Answer
Geoff K.
May 16, 2018

#P(2 < X < 7) = 54.43%#.

Explanation:

The picture is a bit blurry, but it appears as though #X# is the number of calls over a 1.5 minute interval. Thus, #X" ~ Poisson"(lambda)# where #lambda = 1.5 xx 4 = 6.#

Thus, #P(X=x)" "=(e^-6 xx6^x)/(x!)#

Since #X# is discrete, we know

#P(2 < X < 7) = sum_(x=3)^6 P(X = x)#

# = P(X"=3")+P(X"=4")+P(X"=5")+P(X"=6")#

We just need to find these 4 discrete probabilities.

#P(X=3) = (e^-6 xx6^3)/(3!) = 0.0892#

#P(X=4) = (e^-6 xx6^4)/(4!) = 0.1339#

#P(X=5) = (e^-6 xx6^5)/(5!) = 0.1606#

#P(X=6) = (e^-6 xx6^6)/(6!) = 0.1606#

Then

#P(2 < X < 7)#

# = P(X"=3")+P(X"=4")+P(X"=5")+P(X"=6")#

# = 0.0892+0.1339+0.1606+0.1606#

#= 0.5443#
#=54.43%#

Related questions
Impact of this question
2267 views around the world
You can reuse this answer
Creative Commons License