How to find points are area with intergration?
How to do Q 12 and Q13?
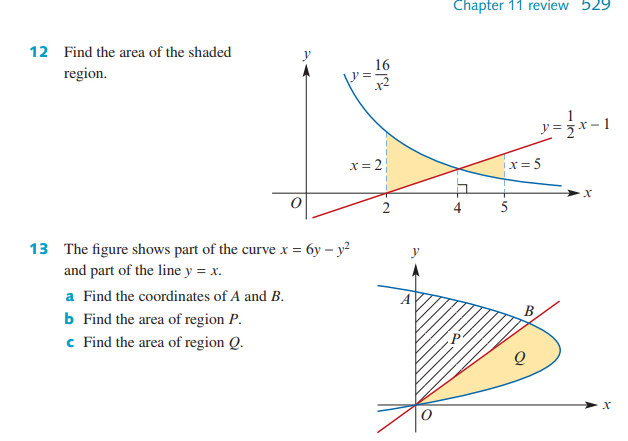
How to do Q 12 and Q13?
1 Answer
12-
13-
a)
b)
c)
Explanation:
Q12

It will help us to write the equation of the curve as
We can use a triangle for the area under the line, but that involves finding points of intersection, and since the line is already in the form
Now for B
Q13
I've rotated the original image to make this a bit easier to see.
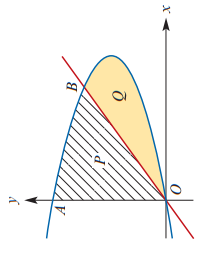
If we were given this, we'd integrate with respect to the horizontal (x) axis, then take away some things and get our area.
We can do exactly the same thing here. This time, instead of integrating with respect to x, we integrate with respect to y. This willl give us the area between the curve and the y axis.
a)
to find A, we need the y-intercepts of the curve.
Let
We can reject
B is the interception between
Solve simultaneously:
Since
b)
The area of P is the area under the curve between A and B, plus the area under the line between B and the origin.
For the area under the line, its easier to treat it like a triangle.
c)
