Please solve q 17?

We assume the quadrilateral has its vertices in alphabetical order (i.e. it can be called #ABCD#).

Since the diagonals of #ABCD# are perpendicular, their intersection creates 4 right triangles inside #ABCD#, and each side of the quadrilateral is the hypotenuse of one of these triangles.

Label the interior sides as #a, b, c, d# as shown below:

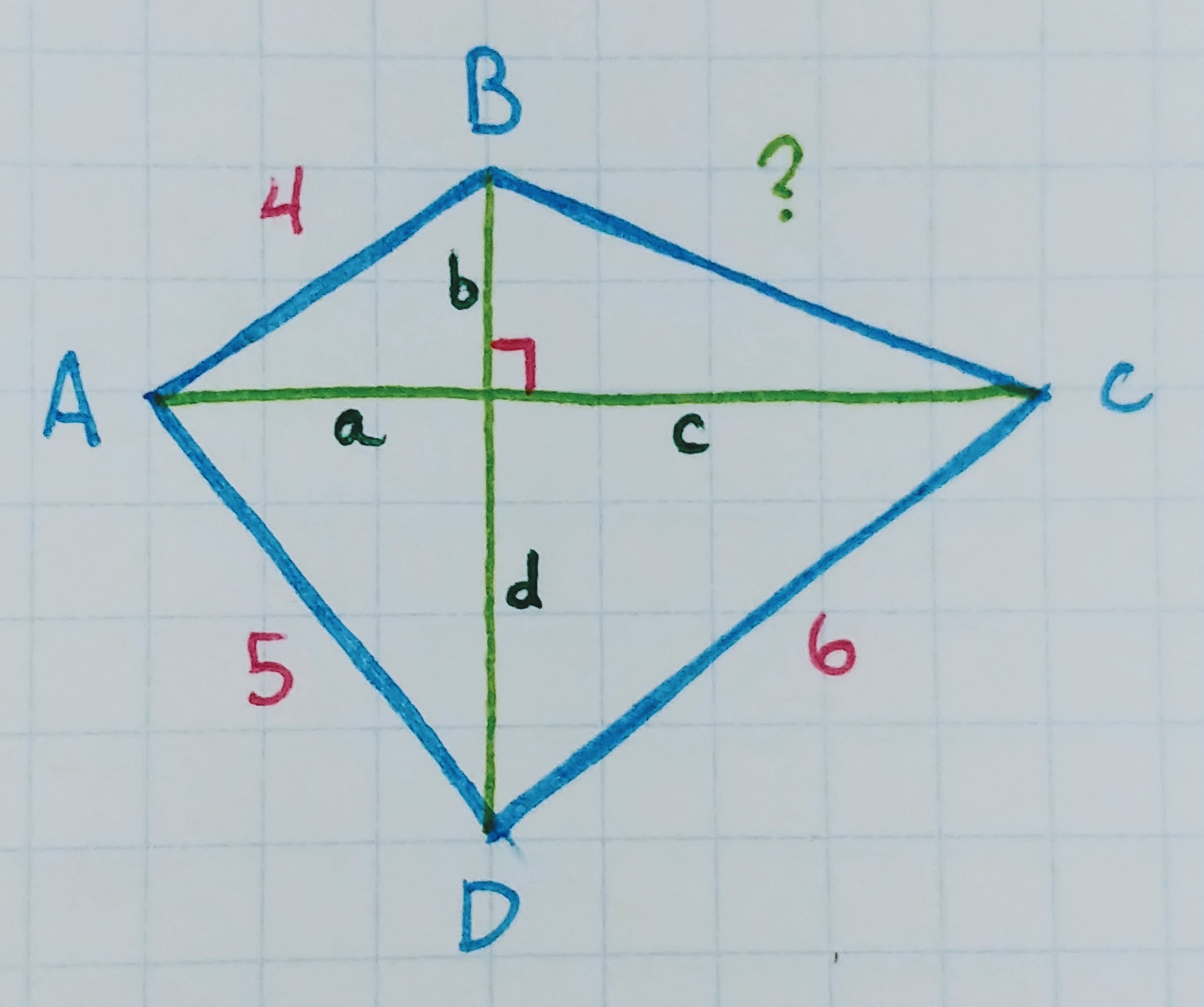
By the Pythagorean theorem, we get the following 4 equations:

(1) #a^2+b^2color(white)("  "+c^2+d^2)=4^2#
(2) #color(white)(a^2+)b^2+c^2color(white)("  "+d^2)=(BC)^2#
(3) #color(white)(a^2+b^2+)c^2+d^2=6^2#
(4) #a^2color(white)("  "+b^2+c^2)+d^2=5^2#

Adding equations (1) + (3) gives

(5) #a^2+b^2+c^2+d^2 = 4^2 + 6^2#

while adding (2) + (4) gives

(6) #a^2+b^2+c^2+d^2 = (BC)^2 + 5^2#

The left-hand sides of (5) and (6) are equal. This means their right hand sides must also be equal:

(7) #4^2+6^2 = (BC)^2 + 5^2#

Equation (7) shows us something very useful: in a convex quadrilateral with perpendicular diagonals, the two pairs of squared opposite sides have equal sums.

We now have an equation where #BC# is the only unknown. We can now solve for #BC#.

#16+36 = (BC)^2 + 25#

#=> (BC)^2 = 16+36 - 25#
#color(white)(=>(BC)^2)=27#

#=>BC = sqrt(27)#
#color(white)(=>BC)=3sqrt(3)#