Two similarly charged spheres A and B separated by a distance 'r' with force of 2×10^-5N. A third identical uncharged sphere C is touched to A and then placed between a mid point of A and B. Calculate net electrostatic force on C?
1 Answer
May 4, 2018
Explanation:
- make a drawing to facilitate the solution
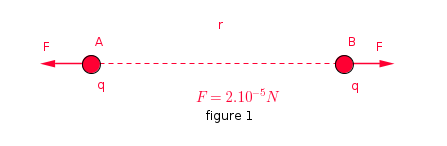
- Coulomb's law can help you calculate the force that two electric charges apply to each other.
-
#F=k*(q_1*q_2)/r^2# -
#color(red)(2*10^-5)=k*(q*q)/r^2=color(red)(k*q^2/r^2)# - The force is directly proportional to the product of the electric charges.
- The force is inversely proportional to the square of the distance between the electric charges.
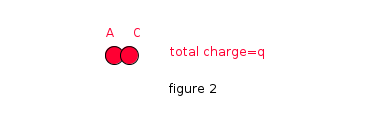
- Since the spheres are identical, the total load is shared equally
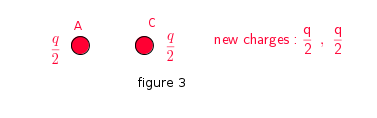
-
The new electric charge distribution of the A and C spheres is shown in Figure 3.
-
Figure 4 shows the new load sequence.
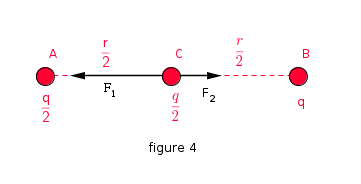
-
C is pushed by both A and B.
-
#F_1=k*(q/2*q/2)/(r/2)^2=k*(q^2/4)/(r^2/4)=color(red)(k*q^2/r^2=2.10^-5)# -
#F_2=k*(q*q/2)/(r/2)^2=k*(q^2/2)/(r^2/4)=2color(red)(k*q^2/4)=color(red)(2*2*10^-5=4*10^-5)# -
Now we must find the vector sum of
#F_1""# and#" " F_2# . -
#vec R=vec F_1 +vec F_2# -
magnitude of net electrostatic force over C is:
-
#-4*10^-5+2*10^-5=-2*10^-5" Newtons"# - The direction to B is considered positive.
-The direction of net electrostatic force is toward A.

